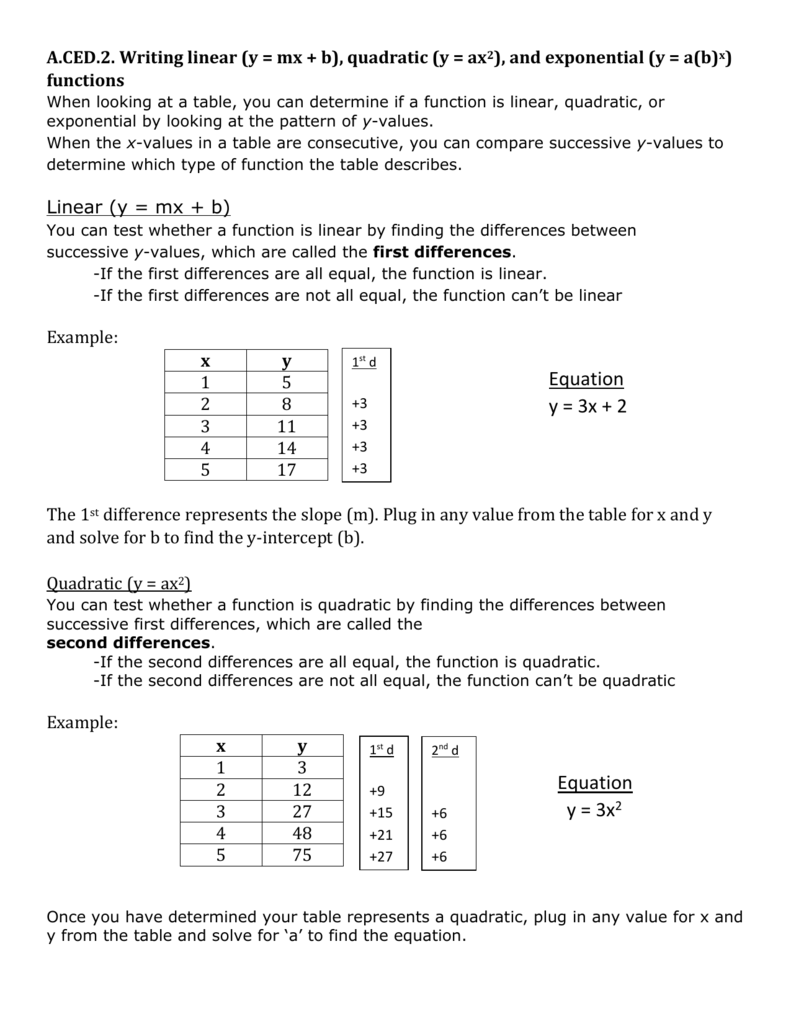
If xy – loge y = 1 satisfies the equation x(yy2 y1^2) – y2 λyy1 = 0, then λ = A –3 B 1 C 3 D none of these asked Apr 15 in Derivatives by Ichha ( 27k points) derivativesLet Y = aX Then, Equation Explanation V(Y) = E(Y2) E(Y)2 = Rule 3 V(X) = E(X E(X))5 = E(X5) E(X)5 = σ5X, ie Definition of the variance E(a2 X2)−E(aX)2 = Substitute for Y Since Y = aX, Y5 = a5X5 a2 E( X2) a2 E(X)2 = Rule 5 E(aX) = a * E(X), ie Expectation of a constant times a variable = The constant times the expectation ofSubstitute the value of a and b in y= ax b to find line of best fit Algorithm for fitting y = ax b 1 Start 2 Read Number of Data (n) 3 For i=1 to n Read X i and Y i Next i 4 Initialize sumX = 0 sumX2 = 0 sumY = 0 sumXY = 0 5 Calculate Required Sum

यद Y Ax 2 Bx C त Dy Dx न क ल
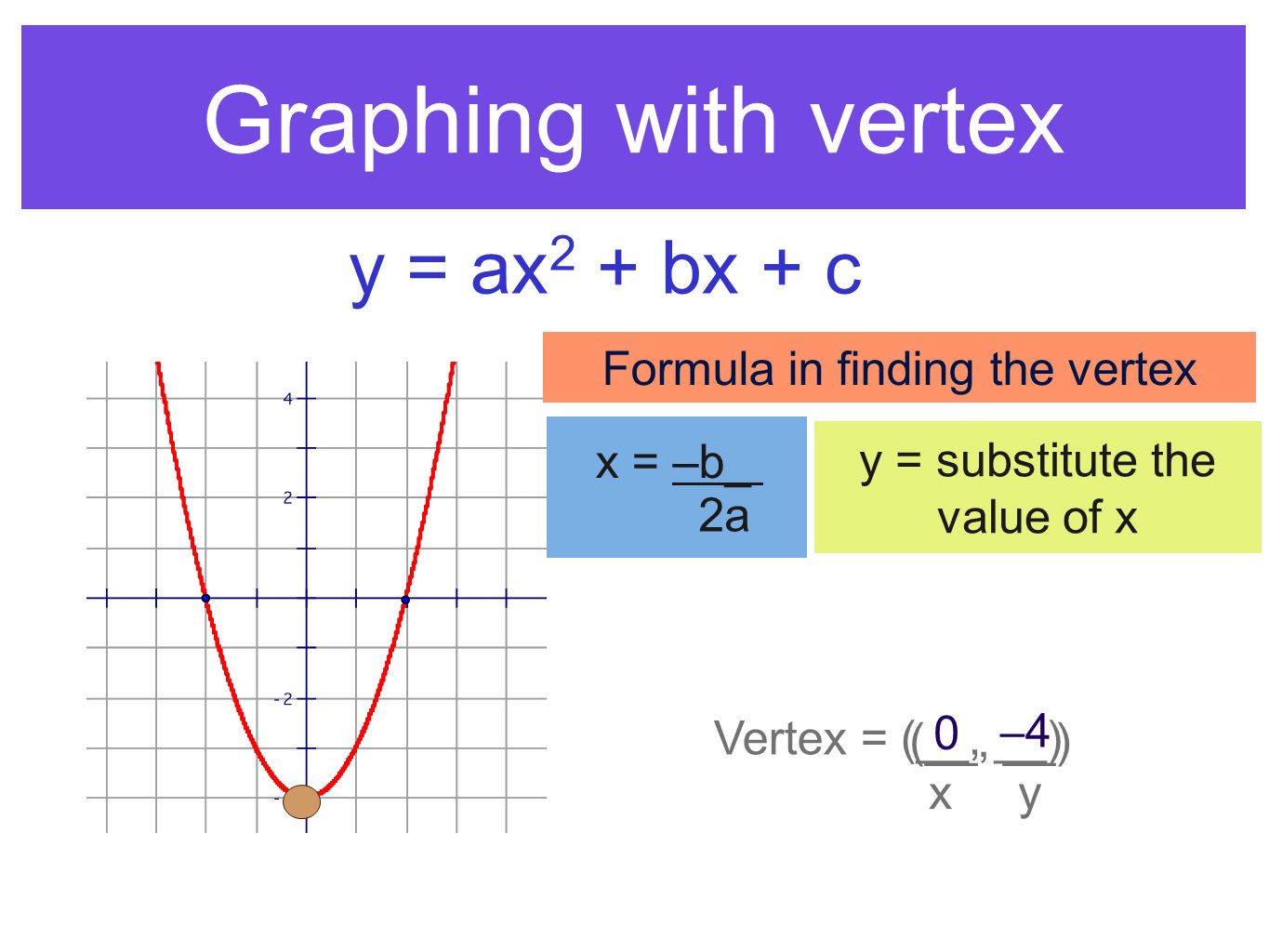
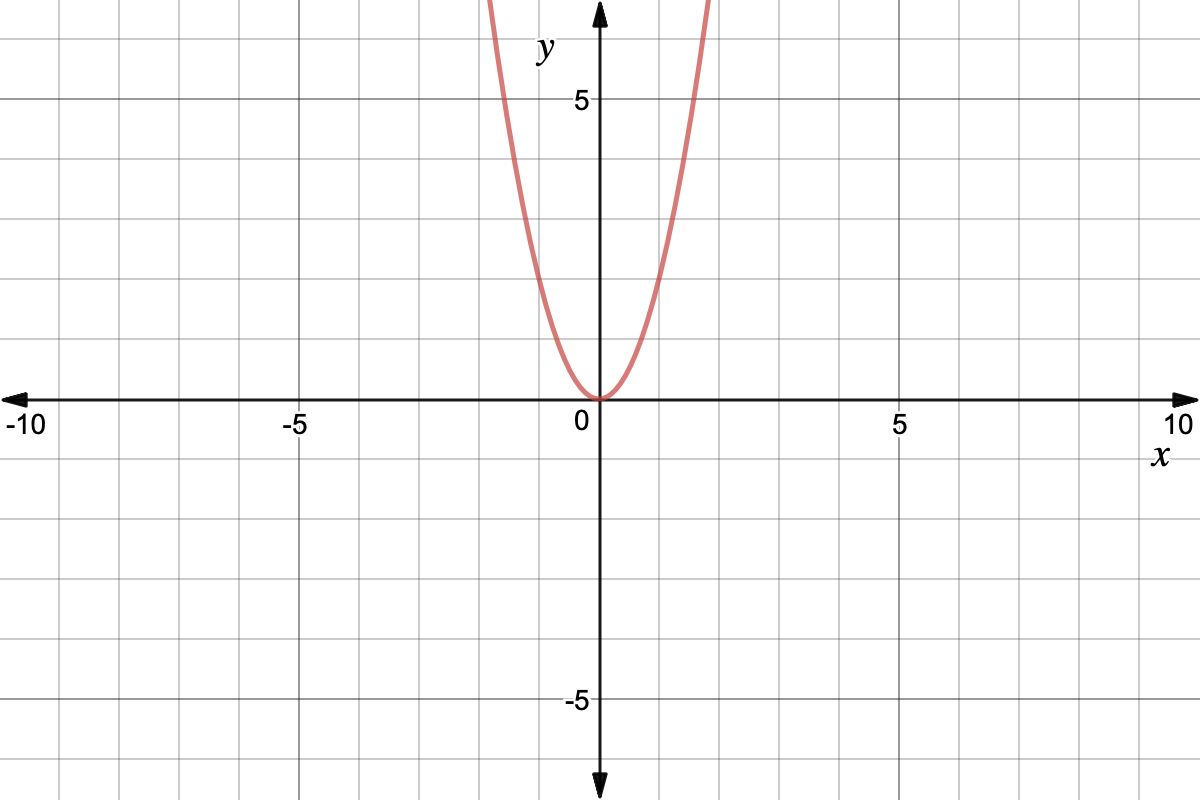
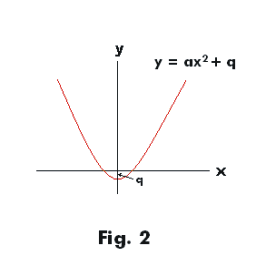
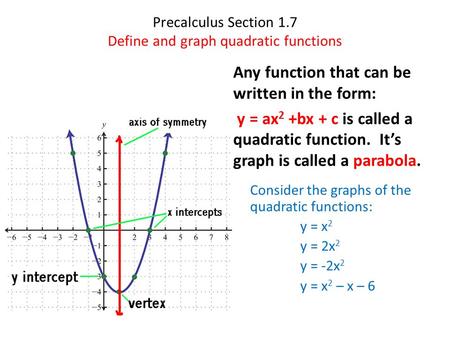
Y=ax^2+c
Y=ax^2+c- 1 Answer The quadratic equation y = ax 2 bx c The above function passes through the points (1,3), (3,1) and (4,0) Solve (1) and (2) to eliminate c variable and obtain two variable equation Solve (2) and (3) to eliminate c variable and obtain two variable equation Solve (4) and (5) to eliminate b variable and obtain one variable About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How works Test new features Press Copyright Contact us Creators



Chapter 10 Section 2 Review Quadratic Function Y Ax 2 Bx Cy 2x 2 3x 1 Symmetry Ppt Download
If y = ax^2/ ( (x – a) (x – b) (x – c)) bx/ ( (x – b) (x – c)) c/ (x – c) 1 then prove that, 1/y dy/dx = 1/x (a/ (a – x) b/ (b – x) c/ (c – x)) ← Prev Question Next Question → 0 votes 197k views asked in Limit, continuity andI hope you enjoy this video, and more importantly, that it helps you out! Stack Exchange network consists of 178 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack Exchange
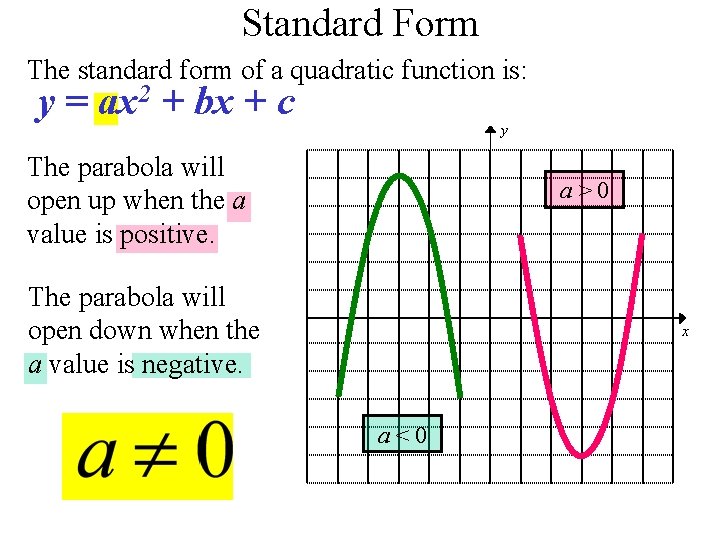
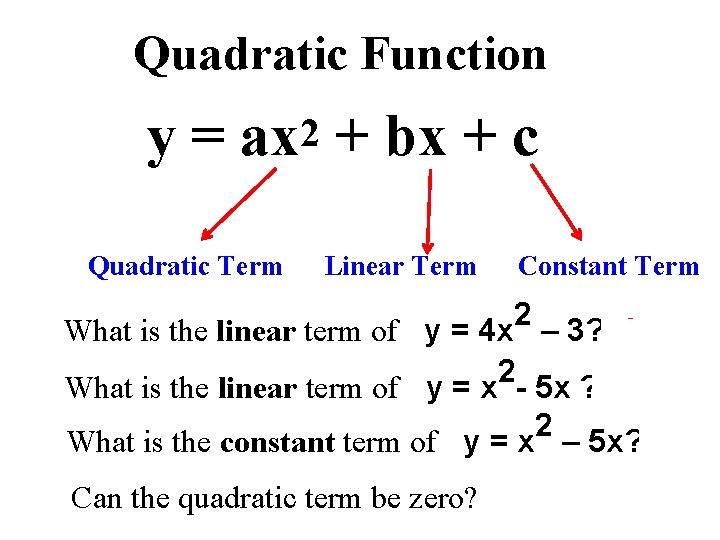
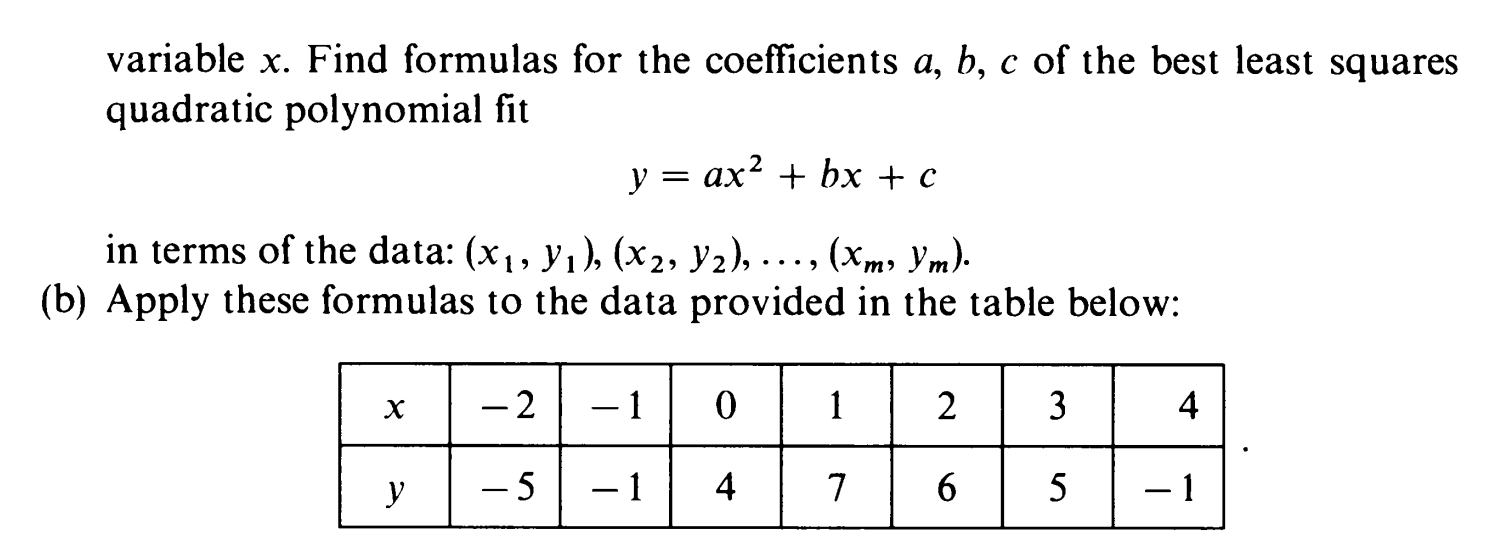
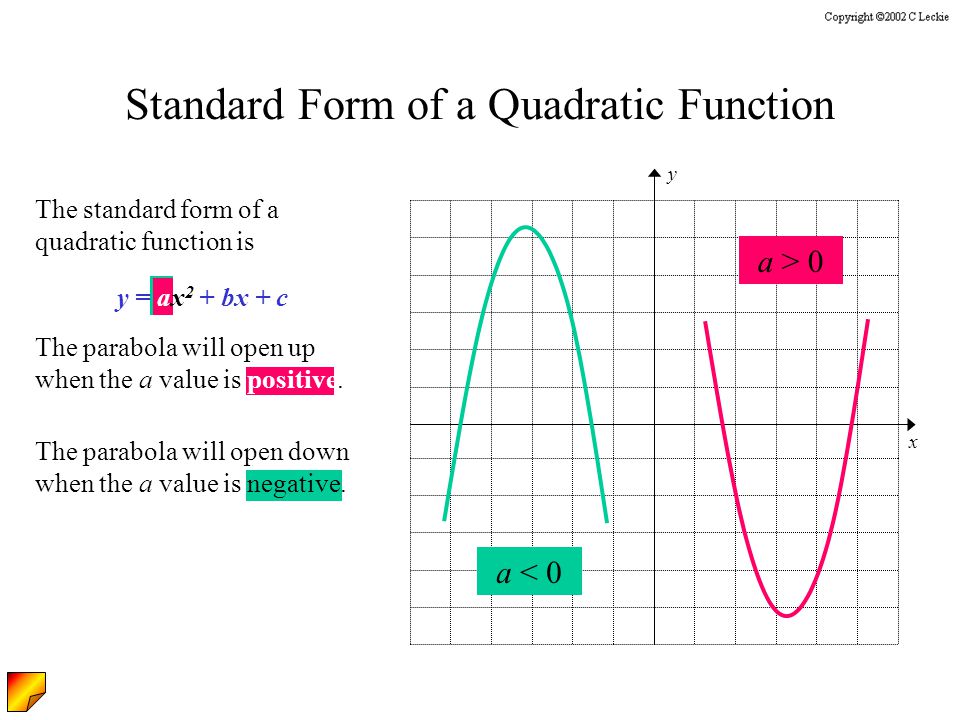
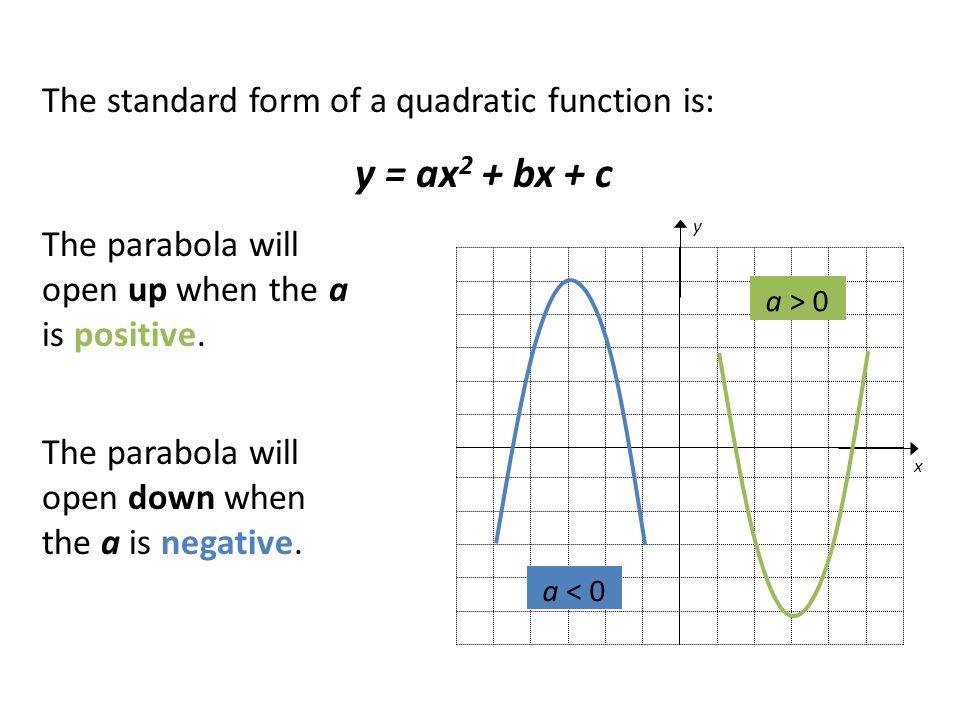
$ y = ax^2 bx c $ The role of 'a' If $$ a > 0 $$, the parabola opens upwards ;Properties A linear function is a polynomial function in which the variable x has degree at most one f ( x ) = a x b {\displaystyle f (x)=axb} Such a function is called linear because its graph, the set of all points ( x , f ( x ) ) {\displaystyle (x,f (x))} in the Cartesian plane, is a line The coefficient a is called the slope of theLearn termquadratics = y = ax^2 bx c with free interactive flashcards Choose from 68 different sets of termquadratics = y = ax^2 bx c flashcards on Quizlet
Find x and y that satisfies ax by = n Print any of the x and y satisfying the equation Examples Input n=7 a=2 b=3 Output x=2, y=1 Explanation here x and y satisfies the equation Input 4 2 7 Output No solution Recommended Please solve it on PRACTICE first, before moving on to the solution We can check if any solutions exists orAnswer to Find the length of the curve y = ax^2 from x = 0 to x = 14, where a greater than 0 is a real number Set up the integral that gives theWe want to put it into vertex form y=a(xh) 2 k;



We Know How To Graph The Quadratic Equation Y X2
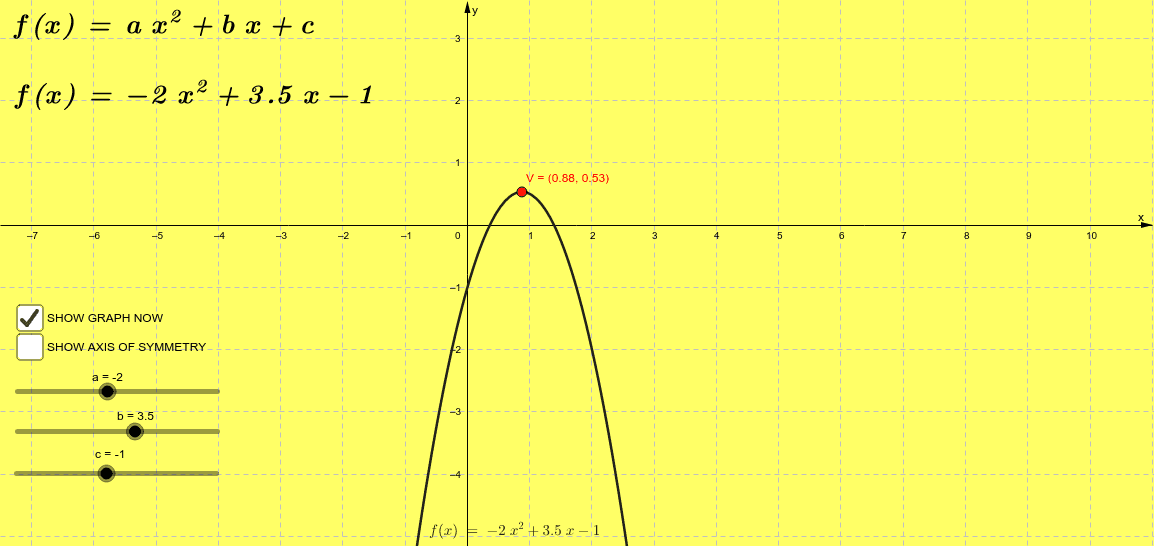



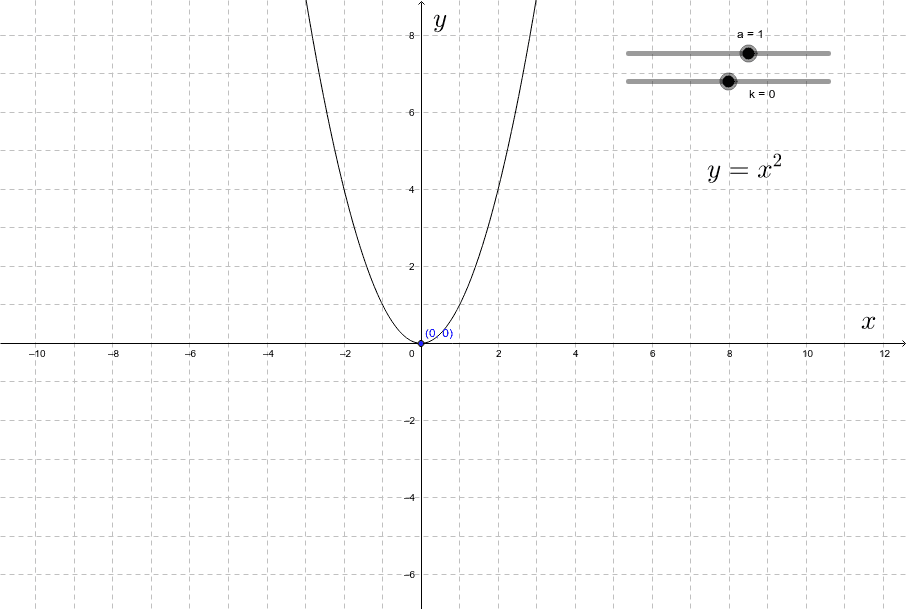
The Quadratic Function Y Ax2 Bx C Geogebra
The parabola has the equation y=2x^2x If y=ax^2bx then y'=2axb This gives us our slope of y at any given x So at the point (1,1), the slope must be y'=2a(1)b=2ab We know the slope must also be 3 at the point (1,1), to match the linear equation given 1 Answer The parabola equation is y=ax^2bxc The point (1,3) passes through parabola so it satisfy the curve The tangent point will also satisfy the parabola So (2,1) will satisfy the curve To find out the tangent , equate the first derivative at (2,1) The given tangent is y = #1 A cubic polynomial function f is defined by f(x) = 4x^3 ax^2 bx k where a, b and k are constants The function f has a local minimum at x = 1, and the graph of f has a point of inflection at x= 2 a) Find the values of Mathfunctions A function, y = f(x), originally has a domain of x equal or bigger than 4 and a range of y equal




Consider The Graphs Of Y Ax 2 And Y 2 3 X 2 4y Where A Is A Positive Constant And X Y In R Number Of Points In Which The Two Graphs Intersect Is



Exploring Quadratic Graphs Ppt Video Online Download
Of the form y = ax 2 The graph is of the form y = ax 2 The given coordinate is ( 2, 1 ) So x = 2 and y = 1 are on the curve Substitute and solve Parabolas of the form y = a(xb) 2 Example Complete the table of values for the equation y= (x2) 2 Plotting these points and joining with a smooth curve gives This time,the graph isThe form y=ax 2 y= x 2 Plot Information Color Xaxis range Minimum Maximum Yaxis range Minimum Maximum Number of points to calculate and plot Quick! The vertex form of a quadratic is given by y = a(x – h) 2 k, where (h, k) is the vertex The "a" in the vertex form is the same "a" as in y = ax 2 bx c (that is, both a's have exactly the same value) The sign on "a" tells you whether the quadratic opens up or opens down




Analyzing The Graph Of Y Ax 2 A 0 Geogebra




Graphing Y Ax 2 C
Something went wrong Wait a moment and try again Try again Please enable Javascript and refresh the page to continueFor an organized list of my math videos, please go to this website https//sitesGiven verbal, graphical, or symbolic descriptions of the graph of y = ax 2 c, the student will investigate, describe, and predict the effects on the graph when "a" is changed TEKS Standards and Student Expectations A(7) Quadratic functions and equations The student applies the mathematical process standards when using graphs of quadratic functions and their related



The Graph Of Y Ax 2 Bx C Has A Minimum At 5 3 And Passes Through 4 0 How Do I Find The Values Of A B And C Quora



Quadratic Function Y Ax 2 Bx C Quadratic
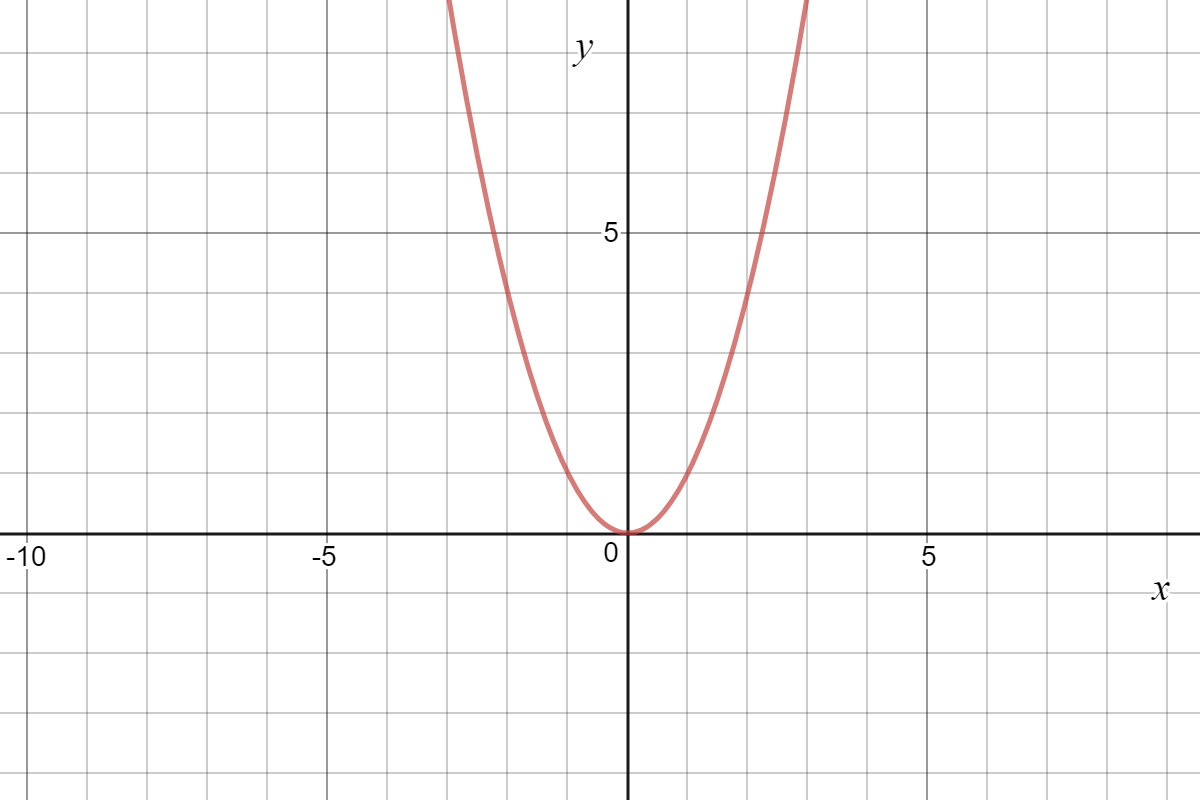
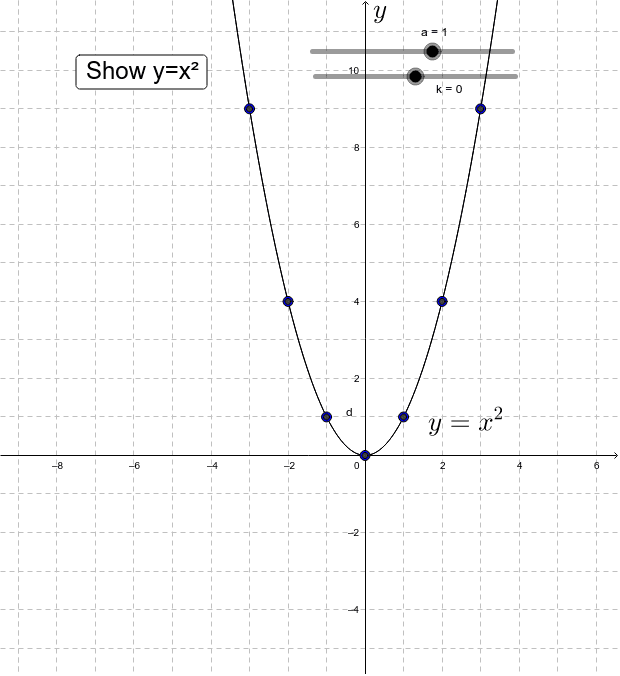
Arguably, y = x^2 is the simplest of quadratic functions In this exploration, we will examine how making changes to the equation affects the graph of the function We will begin by adding a coefficient to x^2 The movie clip below animates the graph of y = nx^2 as n changes between The graph of y=ax^2bxx is given below, where a,b , and c are integers Find abc I assume that is meant to be The graph of y=ax^2bx c is given below, where a,b , and c are integers Find abcGiven y = ax 2 bx c , we have to go through the following steps to find the points and shape of any parabola Label a, b, and c Decide the direction of the paraola If a > 0 (positive) then the parabola opens upward If a < 0 (negative) then the parabola opens downward
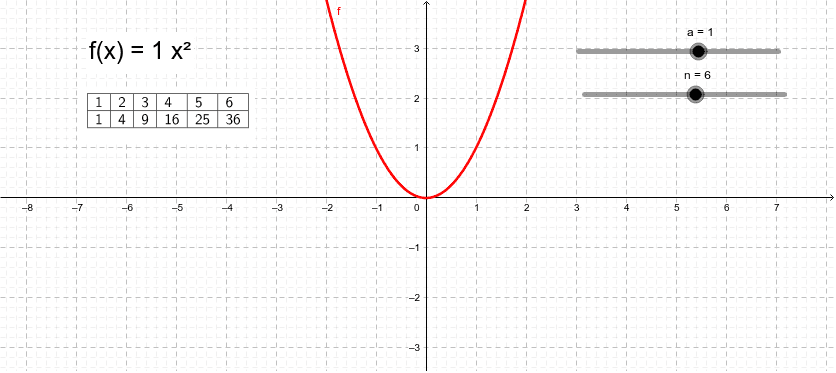



Parabola Y Ax 2 Geogebra




यद Y Ax 2 Bx C त Dy Dx न क ल
Our equation is in standard form to begin with y=ax 2 bxc;If the line 3x y = b is tangential to y = ax^2 at x = 4, the slope of the line is equal to the value of the derivative of y = ax^2 at x = 4 3x y = b => y = 3x b The slope of the tangentIt is known that y = ax^2 bx^3;
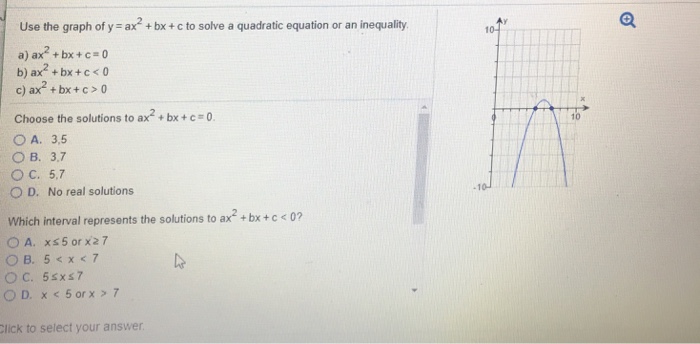



Use The Graph Of Y Ax 2 Bx C To Solve A Chegg Com
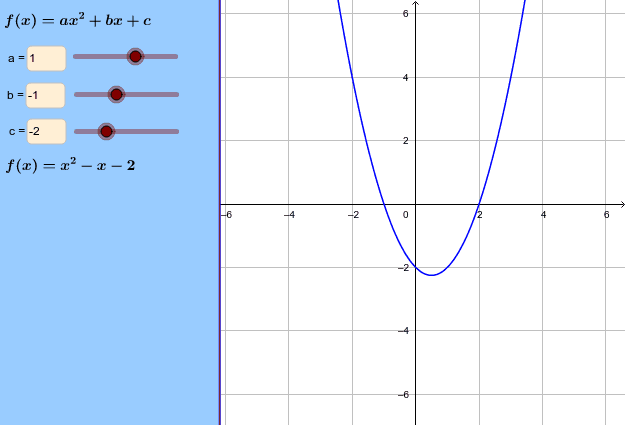



The Graph Of Y Ax 2 Bx C Geogebra
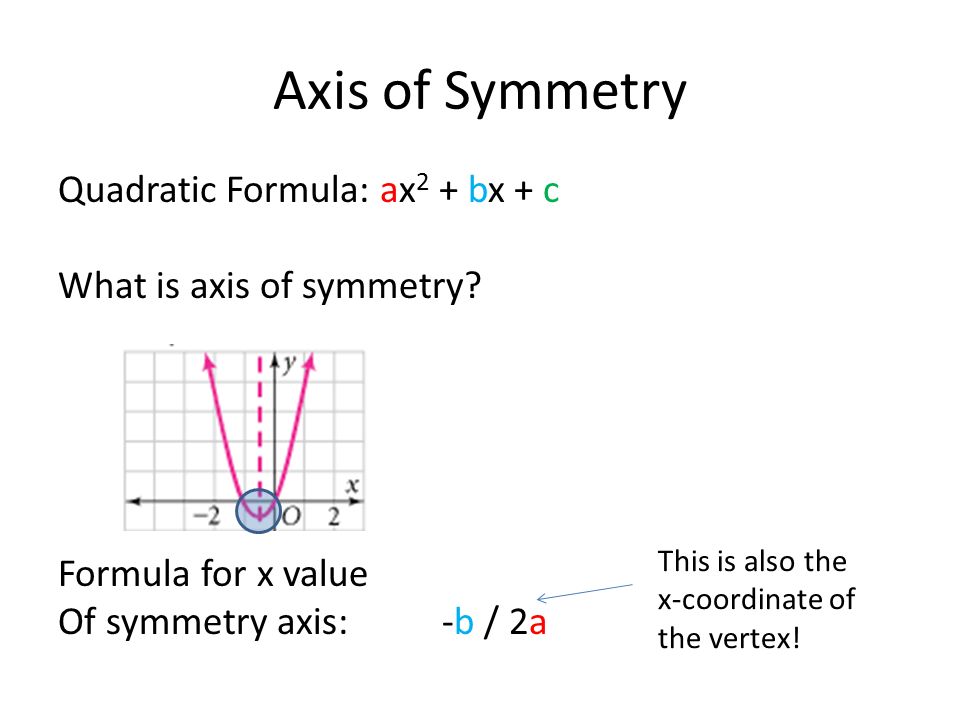
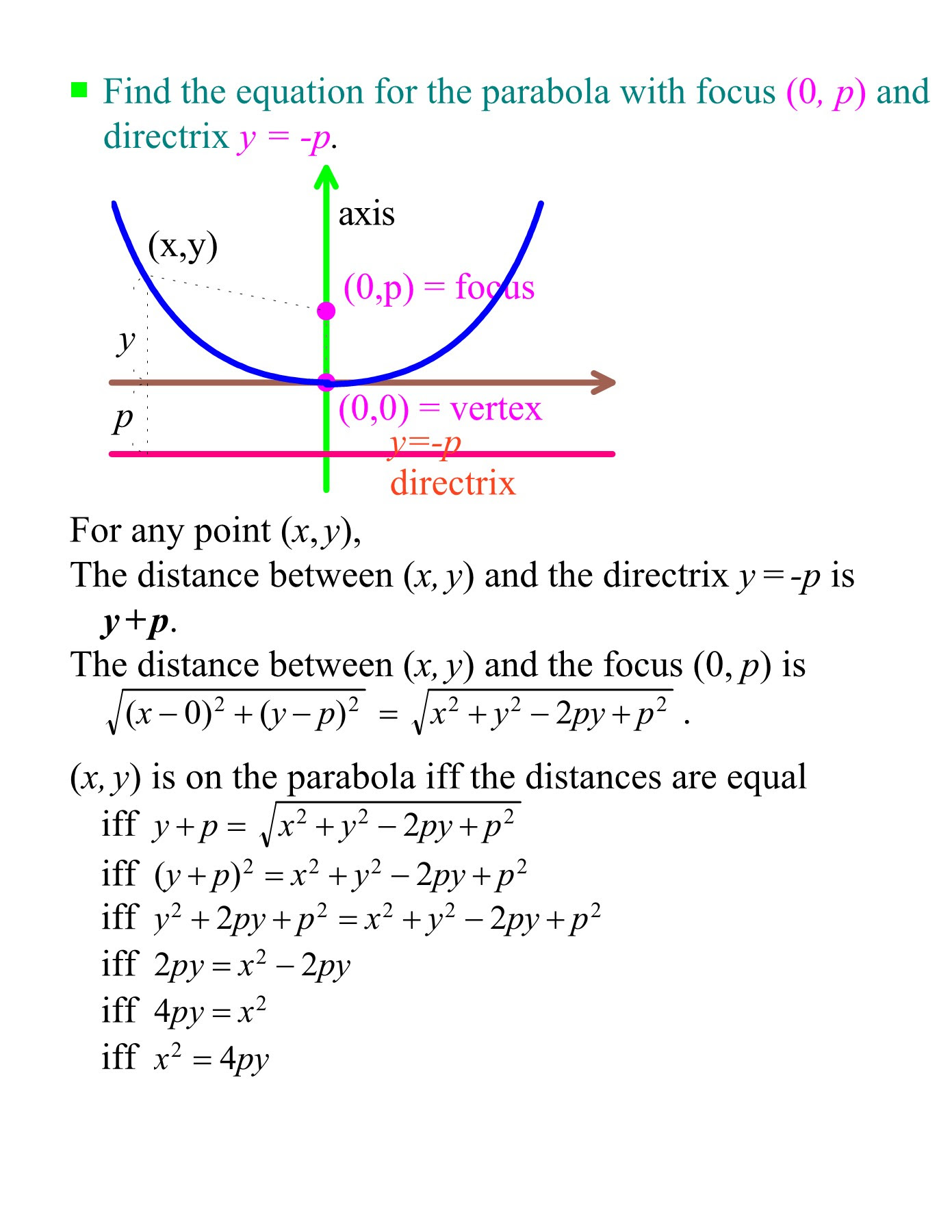
Y = ax 2 bx c or x = ay 2 by c 2 Geometric A parabola is the set of all points in a plane and a given line From the geometric point of view, the given point is the focus of the parabola and the given line is its directrix It can be shown that the line of symmetry of the parabola is the line perpendicular to the directrix through theRewrite the equation as ax2 bx c = y a x 2 b x c = y Move y y to the left side of the equation by subtracting it from both sides Use the quadratic formula to find the solutions Substitute the values a = a a = a, b = b b = b, and c = c−y c = c y into the quadratic formula and solve for x x Simplify the numeratorIf $$ a ;
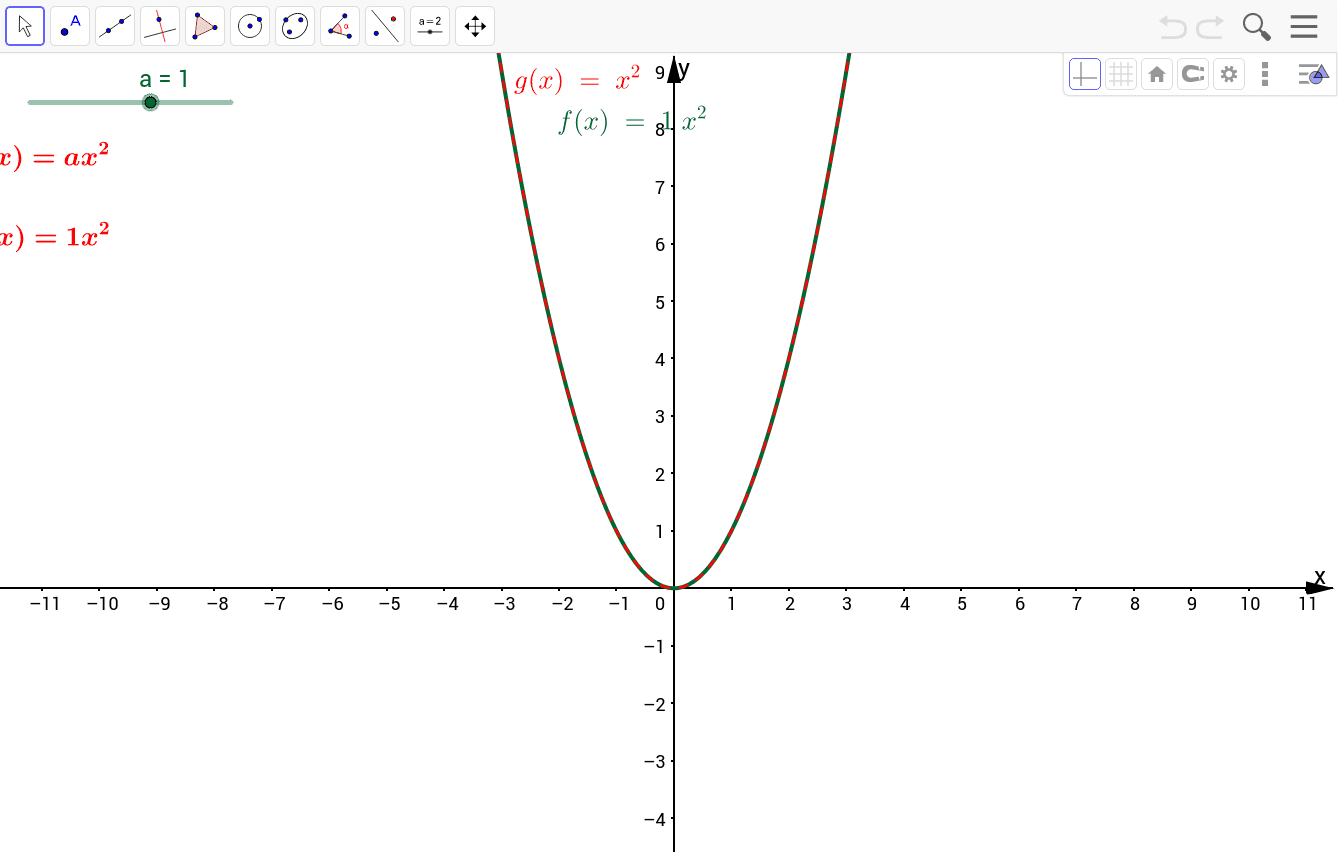



The Properties Of Y Ax 2 Geogebra



Graphing Quadratic Functions Y Ax 2 Bx C
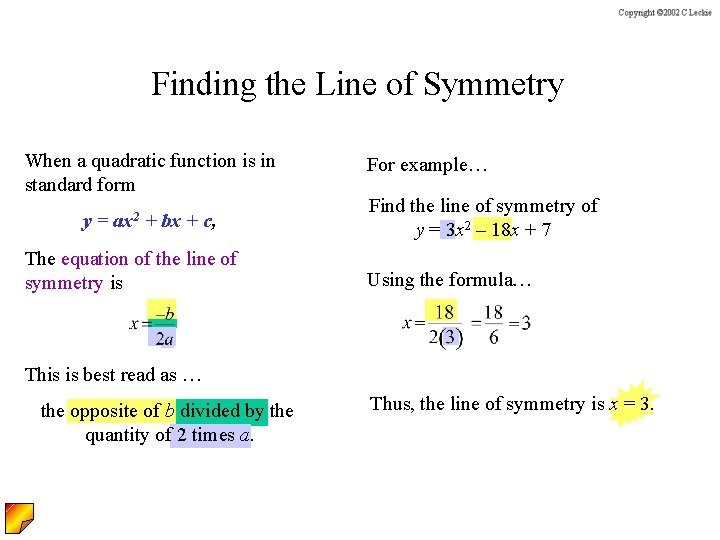
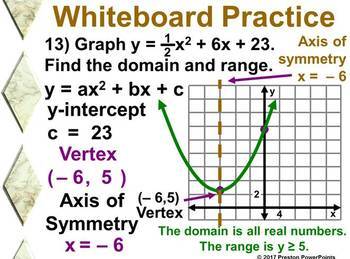
0 $$ it opens downwards The axis of symmetry The axis of symmetry is the line $$ x = \frac{b}{2a} $$ Picture of Standard form equation Axis of Symmetry from Standard Form Vertex Form ofChoose a set of values of y Calculate corresponding x values and plot Draw a smooth curve through the plotted pointsY = ax 2 bx c In this exercise, we will be exploring parabolic graphs of the form y = ax 2 bx c, where a, b, and c are rational numbers In particular, we will examine what happens to the graph as we fix 2 of the values for a, b, or c, and vary the third We have split it up into three parts varying a only varying b only varying c only



Alg 10 1 Graph Y Ax 2 C Youtube




Mbf3c 4 2 The Quadratic Relation Y Ax 2 K Youtube
Find in the form y= ax^2 bx c, the equation of the quadratic whose graph a) touches the xaxis at 4 and passes through (2,12) b) has vertex (4,1) andClick here👆to get an answer to your question ️ If the parabola y = ax^2 6x b passes through (0, 2) and has its tangent at x = 32 parallel to the x axis thenQuadraticequationcalculator ax^2bxc=0 en Related Symbolab blog posts High School Math Solutions – Quadratic Equations Calculator, Part 2 Solving quadratics by factorizing (link to previous post) usually works just fine But what if the quadratic equation



Q Tbn And9gcsf Xgzhrd6b J9wfdp3fg06 Rtae8nem Zikymgw3zmj5rlogv Usqp Cau
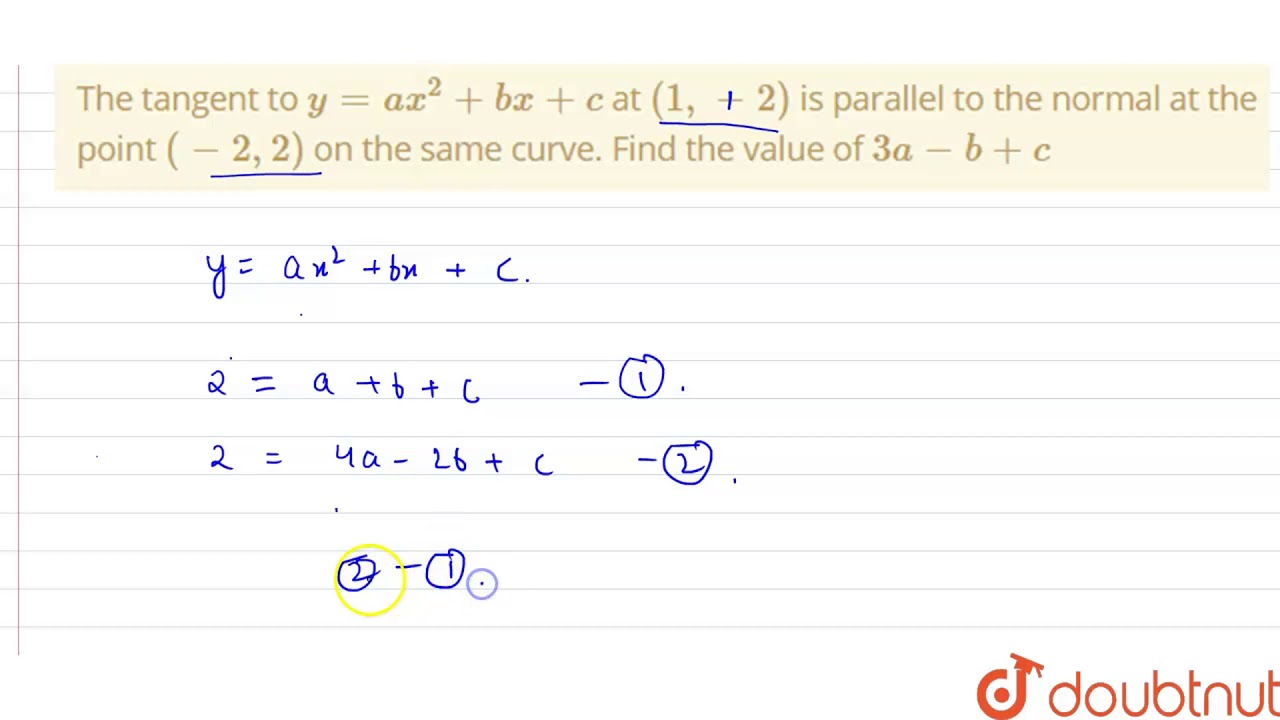



The Tangent To Y Ax 2 Bx C At 1 2 Is Parallel To The Normal At The Point 2 2 On The Youtube
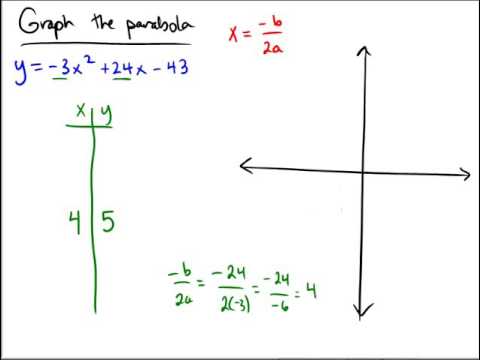
Problem 2 Formula y = ax2 bx c y = x2 4x 8 First we will find the vertex's xcoordinate using –b/2a –b/2a = 4/2(1) = 4/2 = 2 Since 2 is our xcoordinate we will now endeavor to find our ycoordinate y = (2)2 4(2) 8y=4–y = 4, so our vertex is at (2, 4) 17Click here👆to get an answer to your question ️ The area enclosed between the curves y = ax^2 and x = ay^2 (a>0) is 1 squnit then a =${ y = ax^2 bx c \ where \ a \ne 0}$ Least square method can be used to find out the Quadratic Regression Equation In this method, we find out the value of a, b and c so that squared vertical distance between each given point (${x_i, y_i}$) and the parabola equation (${ y = ax^2 bx 2
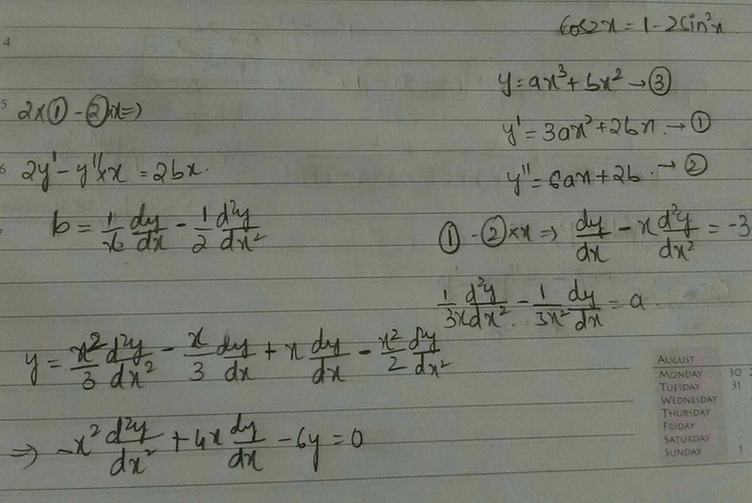



Form Differential Equation Of Y Ax 3 Bx 2 Scholr



Parabola Conic Section Ppt Download
Learn termquadratic equation = y=ax^2bxc with free interactive flashcards Choose from 21 different sets of termquadratic equation = y=ax^2bxc flashcards on QuizletDerive $y=a(xh)^2k$ from $y=ax^2bxc$ given a vertex and a point Recently I have been solving a problem to which I could not find a solutionThere are many differential equations for which mathy(x)=ax^2be^{x}/math is a solution Perhaps the most obvious one is the result of differentiating both side of the equation math\frac{dy}{dx} = 2ax be^{x}/math of course you can the



An Exploration Of The Graph Of Y Ax 2 Font
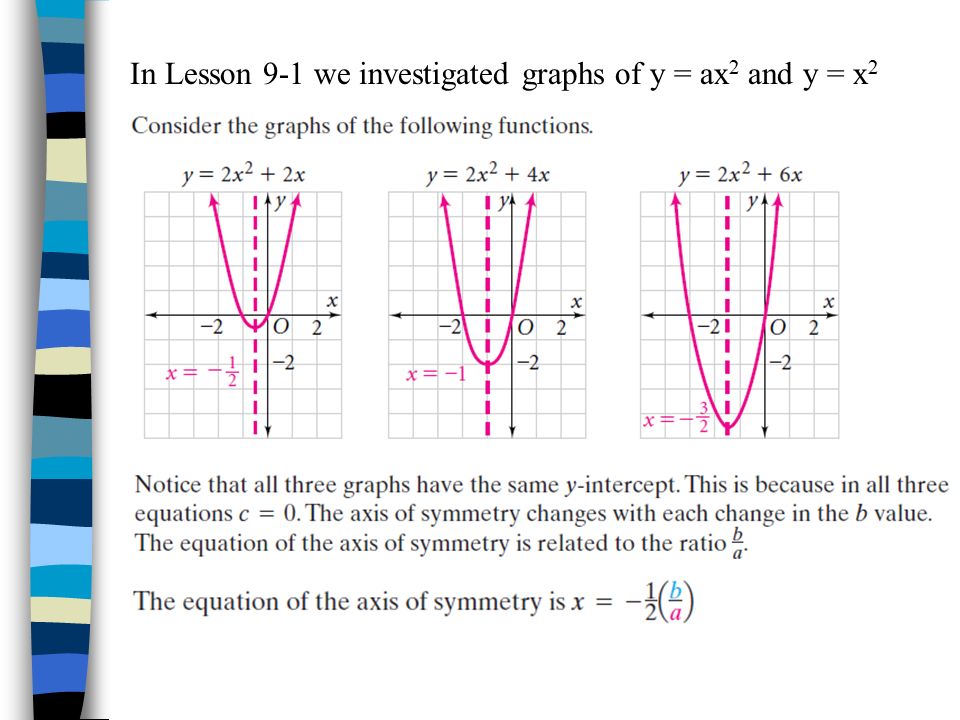



Lesson 9 2 Graphing Y Ax Bx C Objective To Graph Equations Of The Form F X Ax Bx C And Interpret These Graphs Ppt Download
When x = 2, y = 56, and when x = 3, y = 25 Find the values of a and bGiven a quadratic function \(f(x) = ax^2bxc\), it is described by its curve \y = ax^2bxc\ This type of curve is known as a parabola A typical parabola is shown here Parabola, with equation \(y=x^2One Time Payment $1299 USD for 2 months Weekly Subscription $299 USD per week until cancelled Monthly Subscription $9 USD per month until cancelled Annual Subscription $3999 USD per year until cancelled



How Does A Quadratic Graph In The Form Of Y Ax 2 Appear When A Is A Negative Number Quora
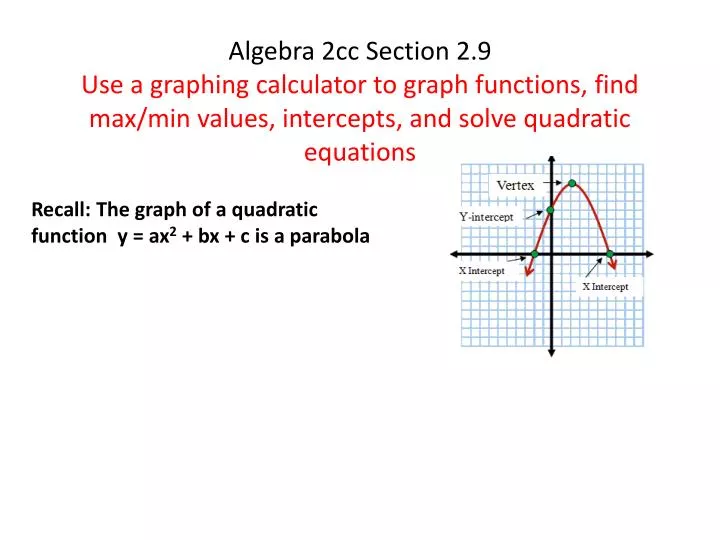



Ppt Recall The Graph Of A Quadratic Function Y Ax 2 Bx C Is A Parabola Powerpoint Presentation Id
Given that the equation of projection is y=axbx^2, we are to find initial velocity(u) and angle of projection(theta) Horizontal component of velocity of projection is ucostheta Vertical component of velocity of projection is usintheta Let at the t th sec after its projection from origin its position be represented by the coordinates (x,y) So x=ucosthetaxxt =>t=x/(ucostheta)1We can convert to vertex form by completing the square on the right hand side;A is the coefficient of the x^2 term In a straight line, the standard form of the equation is ax by = c where a is the coefficient of the x term b is the coefficient of the y term c is the constant term the slopeintercept form of the equation of a straight line is y = mx b where m is the slope b is the y
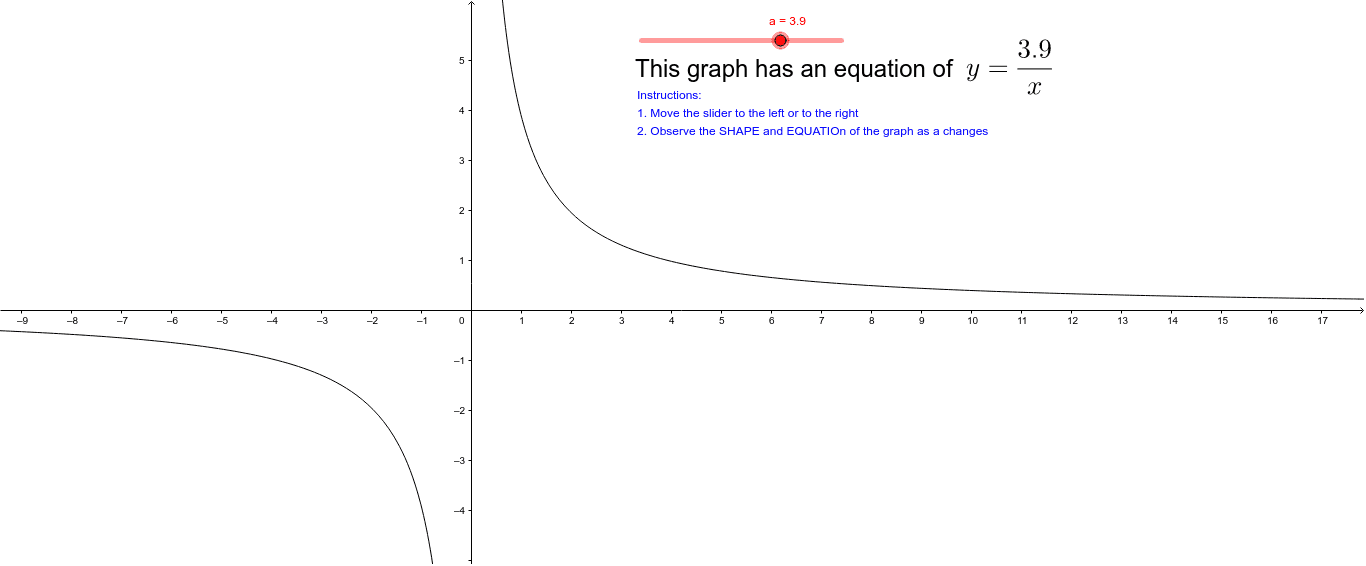



Power Function Graph Y A X Geogebra



Unique Quadratic Equation In The Form Y Ax 2 Bx C
36 is the value for 'c' that we found to make the right hand side a perfect square trinomialGraphing y = ax^2 c 1 Problems0 Problem 1 Graph y = x Problem 2 Graph y = 2x Problem 3 Graph y = ½x Problem 4 Graph y = x Problem 5 Graph y = x2 40 Problem 6 Graph y = x2 Problem 7 Graph y = 2x2 4 2 Problem 10 Graph y = x2 3 Problem 10 Graph y = x2The first thing we need to do is to remember the x and ytableGiven equation y^2 = Note that the origin (0,0) is a point on the graph Graph is symmetric about x axis (Both y and y are mapped to the same x value) To draw the graph, Choose a value for a;



Graph Of Y Ax 2 K Geogebra



Quadratic Graph Example Y Ax Expii
Below you can see the graph of $y=x^26x$ The axis of symmetry of this parabola is the line $$x = {b}/{2a} = {(6)}/{2(1)} = 6/2 = 3$$ We want to find the vertex of this parabola The vertex isBx=yax^{2}c Subtract c from both sides bx=ax^{2}yc Reorder the terms xb=ax^{2}yc The equation is in standard form \frac{xb}{x}=\frac{ax^{2}yc}{x} Divide both sides by x b=\frac{ax^{2}yc}{x} Dividing by x undoes the multiplication by x Examples Quadratic equationCalculus Find dy/db y= (axb)^2 y = (ax b)2 y = ( a x b) 2 Differentiate both sides of the equation d db(y) = d db((axb)2) d d b ( y) = d d b ( ( a x b) 2) The derivative of y y with respect to b b is y' y ′ y' y ′ Differentiate the right side of the equation Tap for more steps



Graphing Quadratic Functions Y 2 Ax Bx C




If Graph Of Y Ax 2 Bx C Is As Shown Then Which Of The Following Is Correct Where D Equals B 2 4ac



Graphing Quadratic Functions Ma 912 A 7 1



Graphing Y Ax 2 Bx C




Graphing A Parabola Of The Form Y Ax2 Bx C With Integer Coefficients Youtube




Graphing Y Ax 2 C
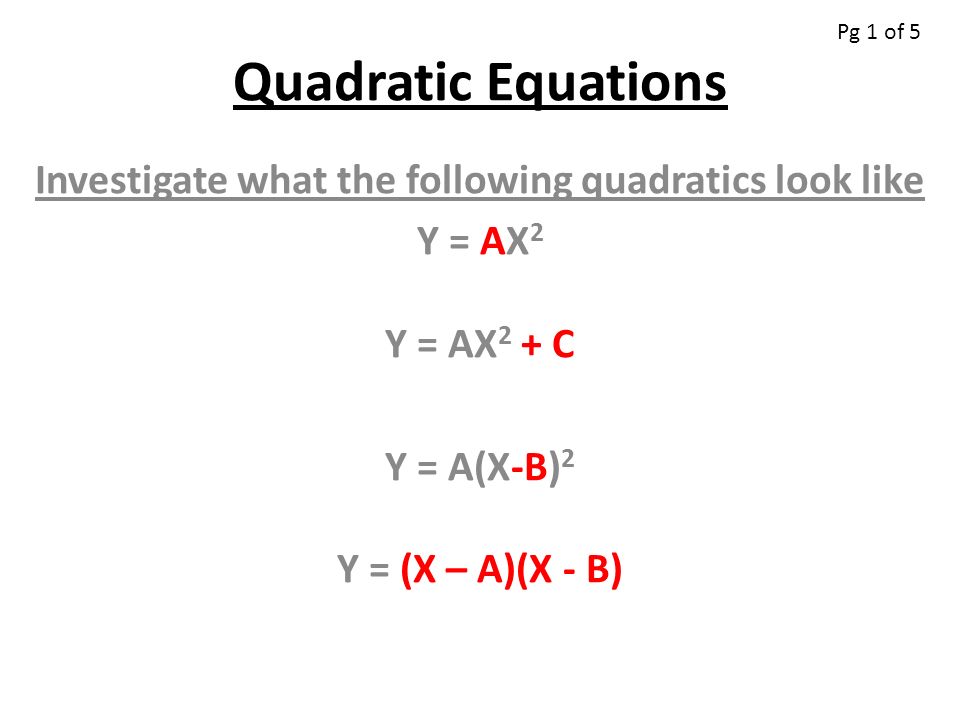



Quadratic Equations Investigate What The Following Quadratics Look Like Y Ax 2 Y Ax 2 C Y A X B 2 Y X A X B Pg 1 Of Ppt Download
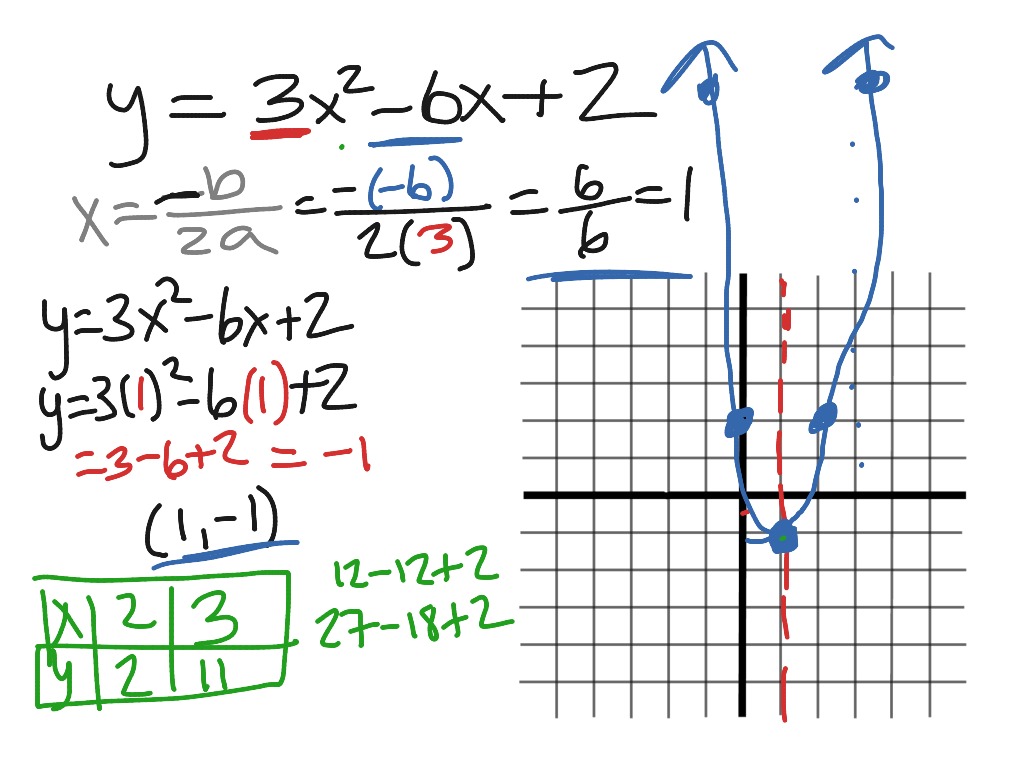



10 2 Graph Ax 2 Bx C Math Showme
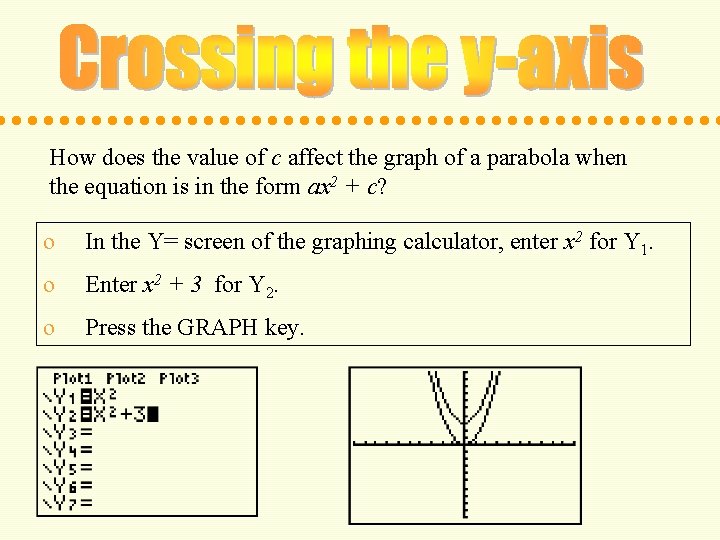



Quadratic Equation Equation In The Form Yax 2
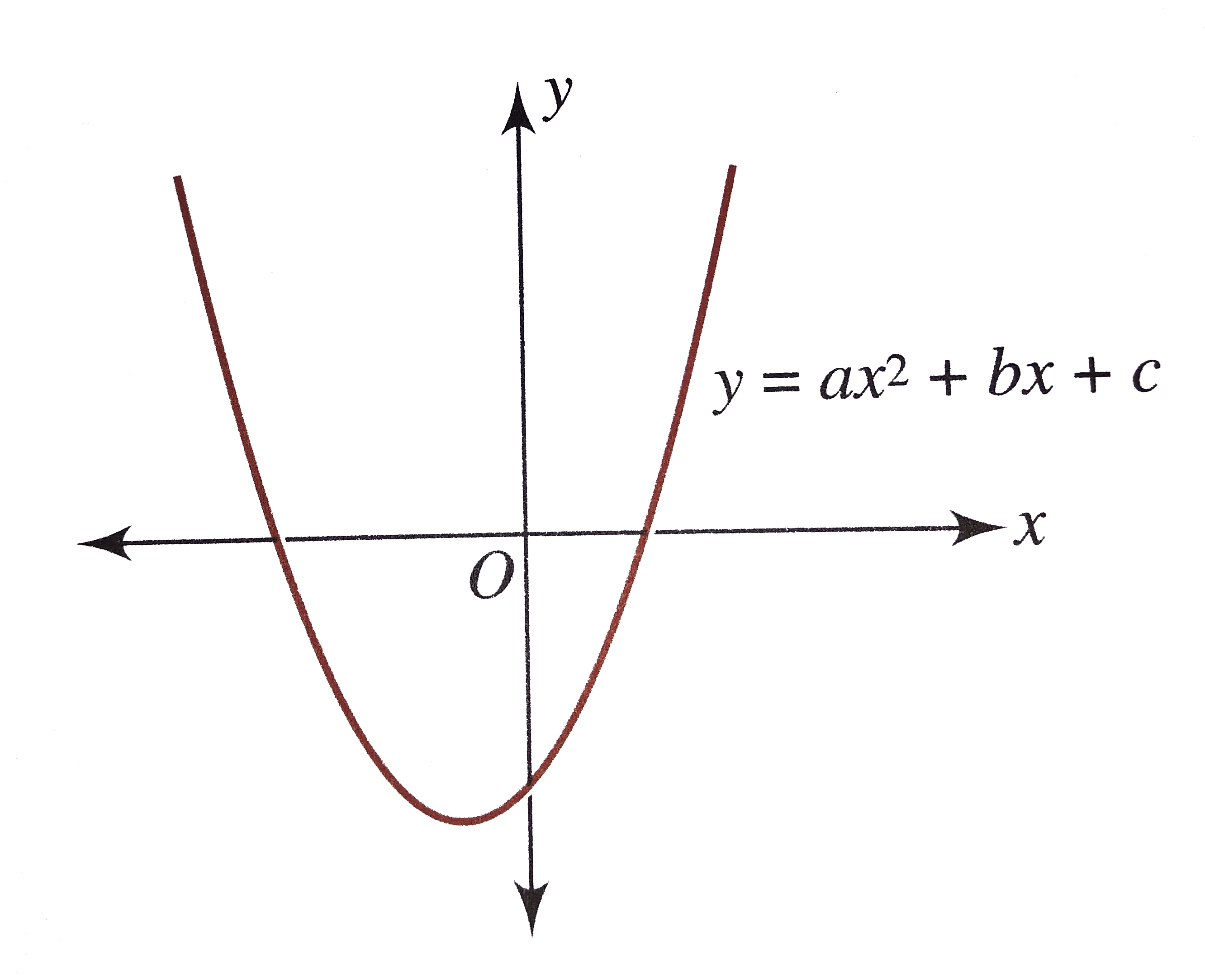



For Following Graphs Of Y Ax 2 Bx C With A B C C R Comment On The Sign Of




Algebra1 Graphing Y Ax 2 Youtube




Clockwise Markers For The Functions Linear Y Ax B Quadratic Y Download Scientific Diagram



Y Ax 2
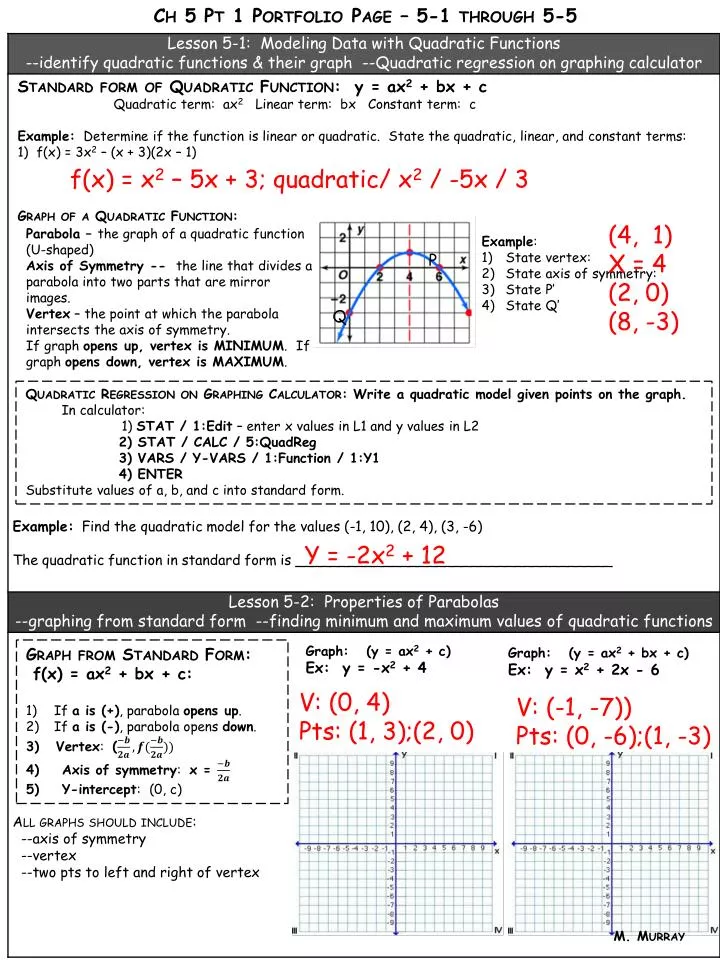



Ppt Standard Form Of Quadratic Function Y Ax 2 Bx C Powerpoint Presentation Id



8 A Suppose That The Variable Y Is Known To Be A Chegg Com



ベストコレクション Yax2 B ニスヌーピー 壁紙



Suppose A Parabola Y Ax 2 Bx C Has Two X Intercepts One Positive And One Negative And Its Vertex Is 2 2 Sarthaks Econnect Largest Online Education Community




Act Math Practice Question 1184 Answer And Explanation Crackact Com



Y Ax 2
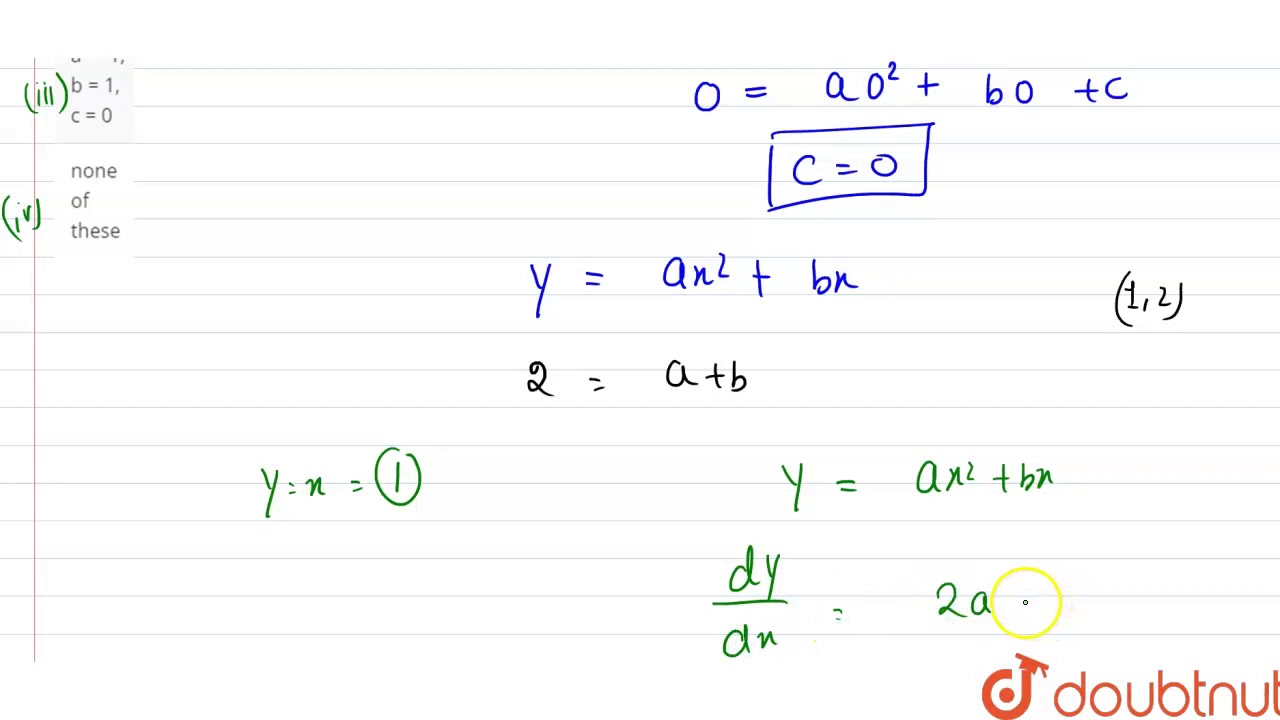



If The Curve Y Ax 2 Bx C Passes Through The Point 1 2 And The Line Y X Touches Youtube




0101 Graphing Quadratic Functions
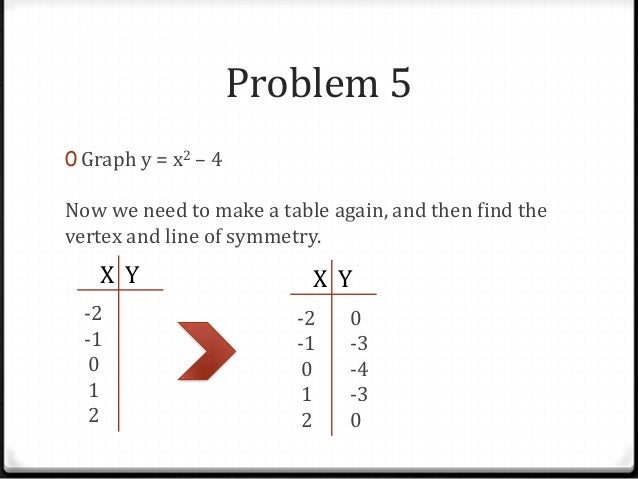



Graphing Y Ax 2 C




Chapter 10 Section 2 Review Quadratic Function Y Ax 2 Bx Cy 2x 2 3x 1 Symmetry Ppt Download
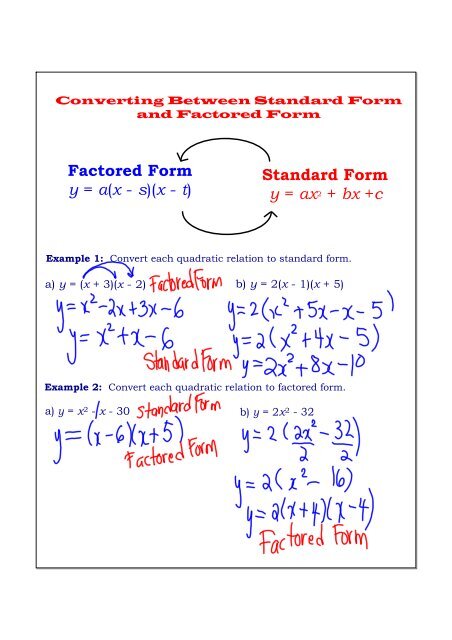



Standard Form Y Ax2 Bx C Factored Form Y A X S X T
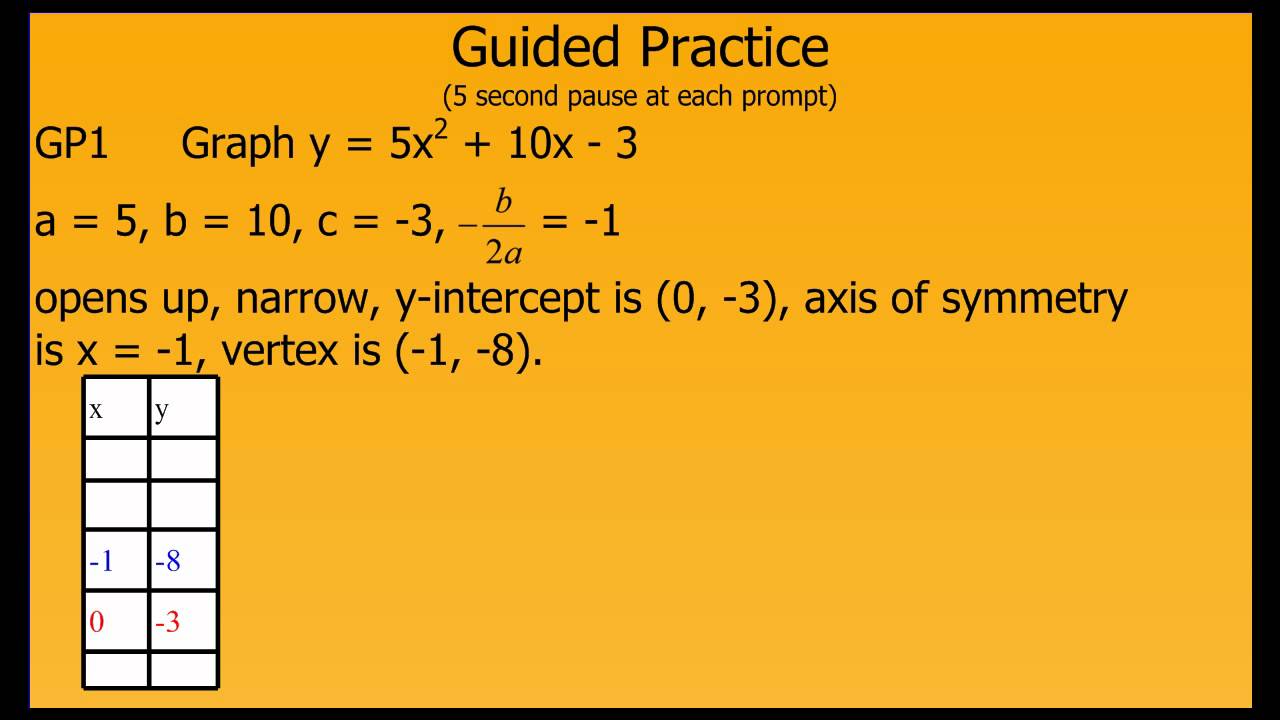



Graphing Y Ax2 Bx C Youtube



Graphing A Parabola Of The Form Y Ax 2 Bx C Integer Coefficients Youtube




Quadratic Function




2 Graph Of Y Ax 2 Bx K Graph Of Y Mx K Download Scientific Diagram



Alg 1 Graphing Y Ax2 Bx C In A Powerpoint Presentation Tpt
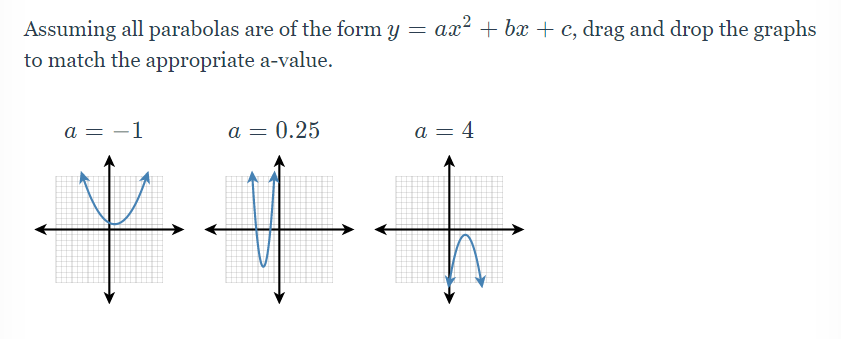



Answered Assuming All Parabolas Are Of The Form Bartleby



Quadratic Graph Example Y Ax C Expii



The Function F X Y And Associated Matrices E And E



Lesson 10 2 Quadratic Functions And Their Graphs Y Ax 2 Bx C Ppt Download
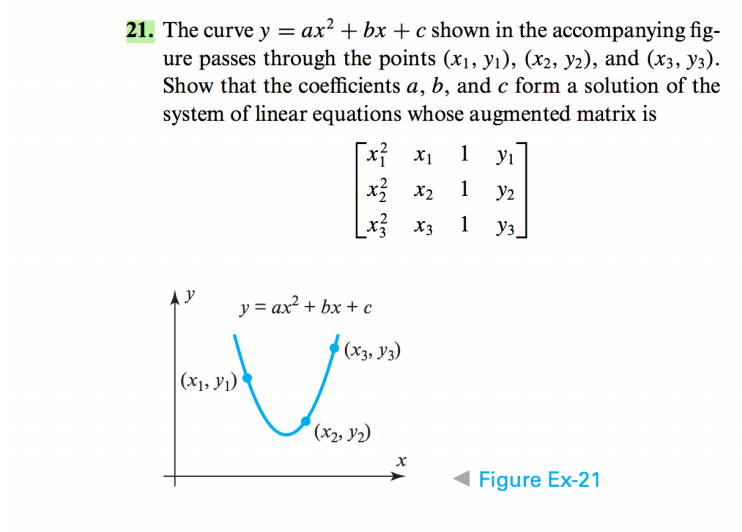



The Curve Y Ax 2 Bx C Shown In The Accompanying Chegg Com



Show That Y Ax 2 Bx C A 0 Represents A Parabola And Find Its Vertex Focus Directrix And Latus Rectum Sarthaks Econnect Largest Online Education Community




This Is What The Problem Says Assuming All Parabolas Are Of The Form Y Ax 2 Bx C Drag And Drop The Brainly Com
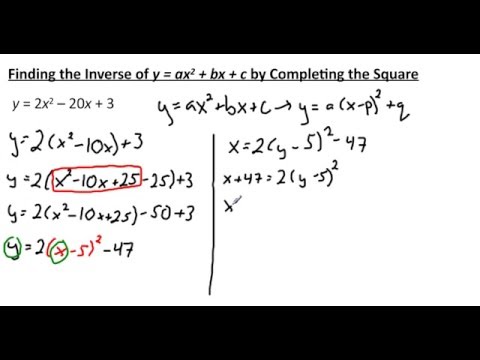



Finding The Inverse Of Y Ax2 Bx C By Completing The Square Youtube



Graphing Y Ax 2 C Activity Builder By Desmos
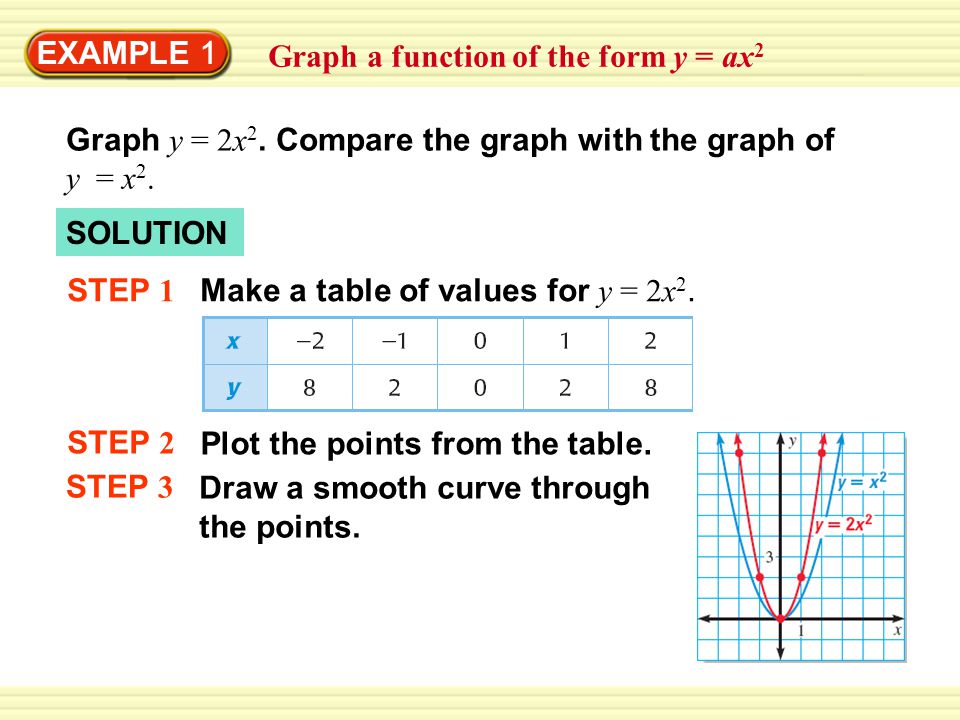



Example 1 Graph A Function Of The Form Y Ax 2 Graph Y 2x 2 Compare The Graph With The Graph Of Y X 2 Solution Step 1 Make A Table Of Values For Ppt Download
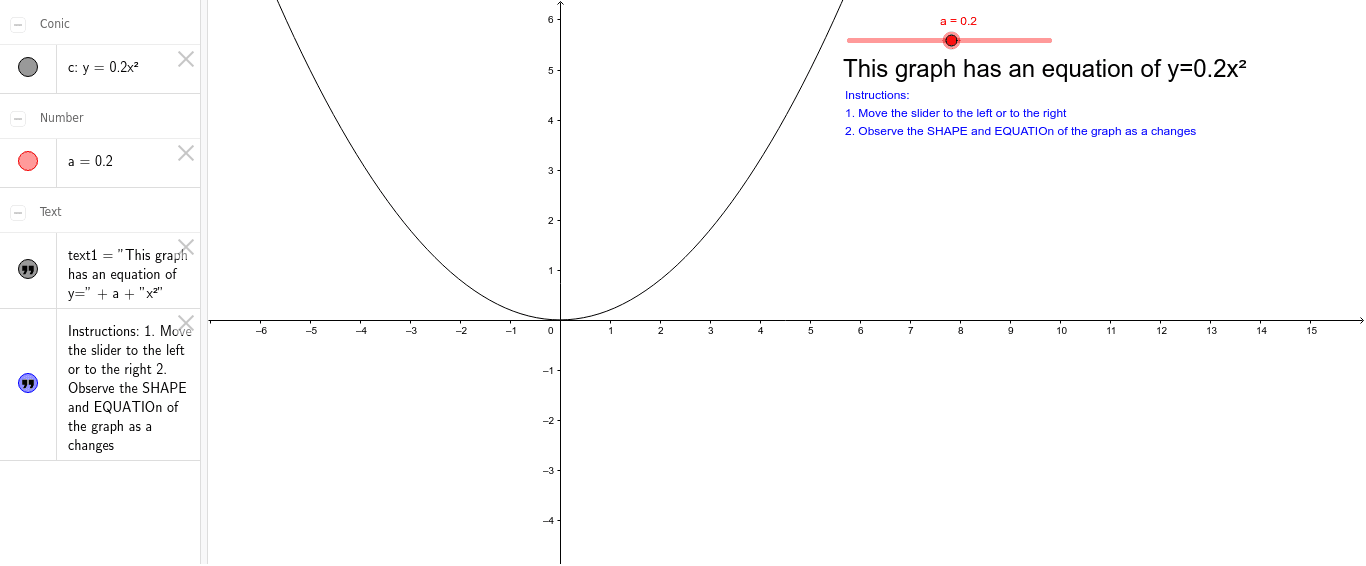



Power Function Graph Y Ax 2 Geogebra




Snapshot Of A Sim Lesson Showing The Graph Of Y Ax 2 Download Scientific Diagram



1
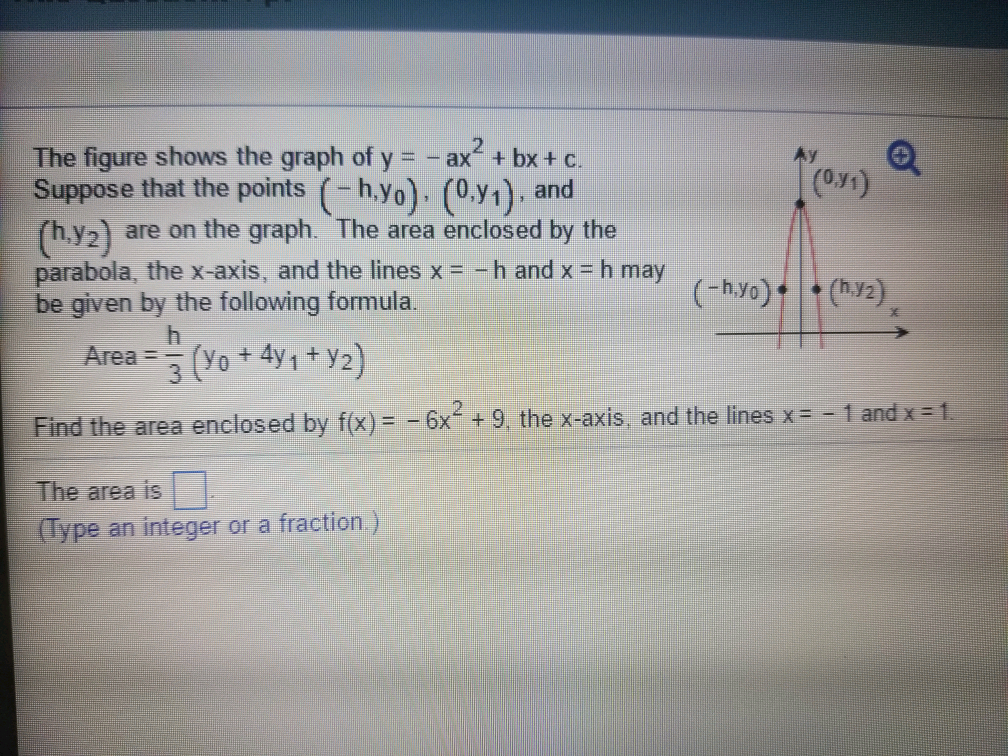



The Figure Shows The Graph Of Y Ax 2 Bx C Chegg Com




Sketching Graphs Of The Form Y Ax2 Q Functions I
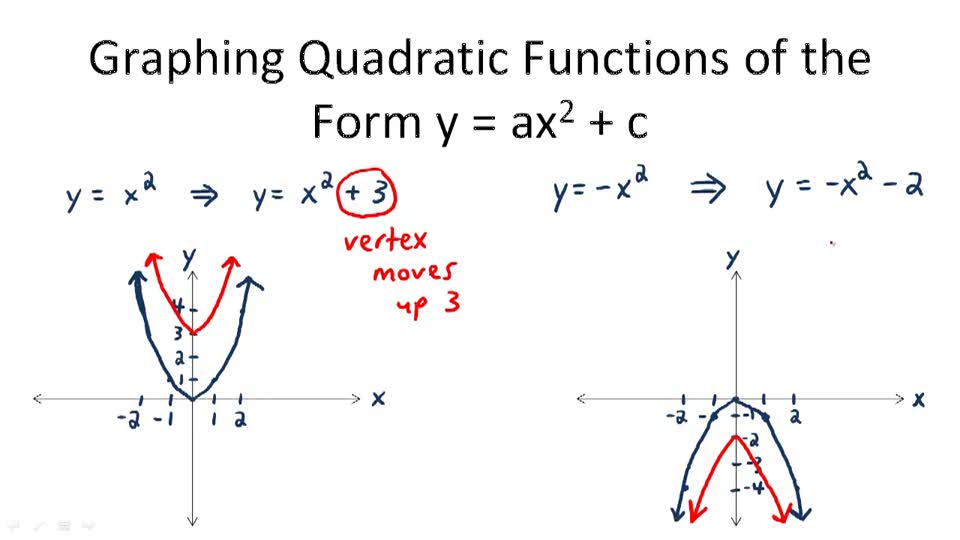



Graphing Quadratic Functions Of The Form Y Ax 2 C Overview Video Algebra Ck 12 Foundation



9 3 Graphing Y Ax Bx C 2 1a Y X 1 For 3 X 3 2 X Y B Does It Open Up Or Down C Identify The Axis Of Symmetry And Its Vertex Ppt Download



Quadratic Graph Example Y Ax C Expii



1



Algebra 1 Activity Sheet On Graphing Y Ax 2 C By Math Through Discovery Llc



Graph Of Y Ax 2 K Geogebra




Graphing Y Ax 2 C
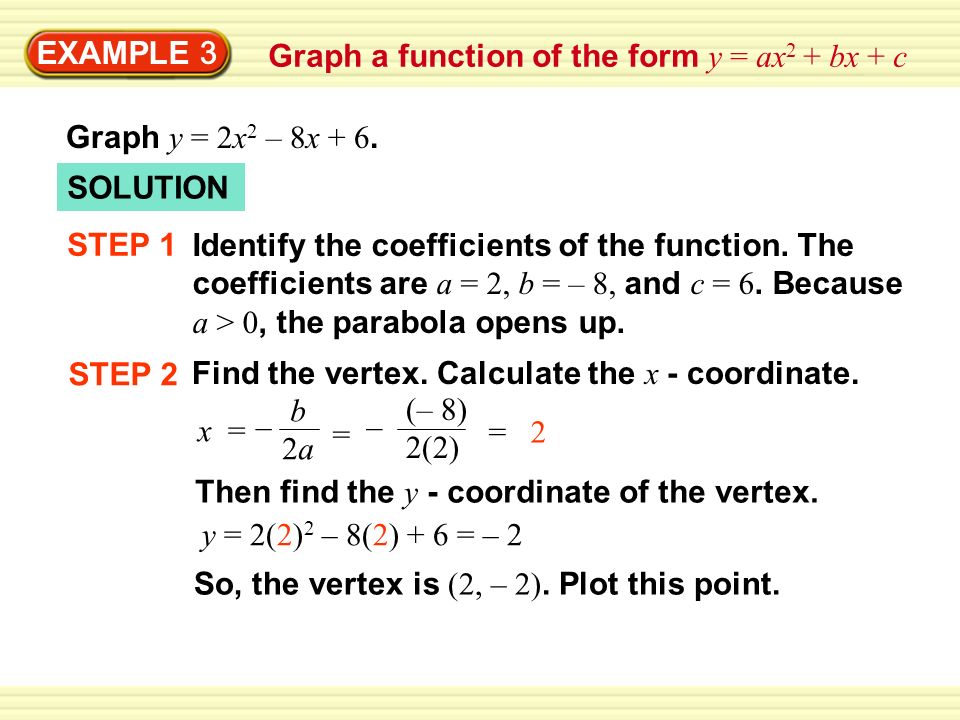



Example 3 Graph A Function Of The Form Y Ax 2 Bx C Graph Y 2x 2 8x 6 Solution Identify The Coefficients Of The Function The Coefficients Ppt Download




Use The Graph Of Y Ax2 Bx C To Solve A Chegg Com



Power Function Graph Y A X 2 Geogebra




If The Curve Y Ax 2 Bx C Passes Through The Point 1 2 And The Line Y X Touches It At The Origin Then




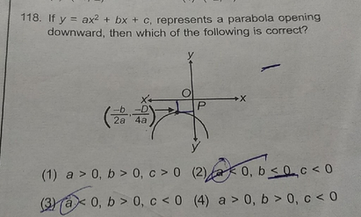



118 If Y Ax2 Bx C Represents A Parabola Opening Downward Scholr



1



Assignment 2



Graphing Parabolas In The Form Y Ax 2 Youtube
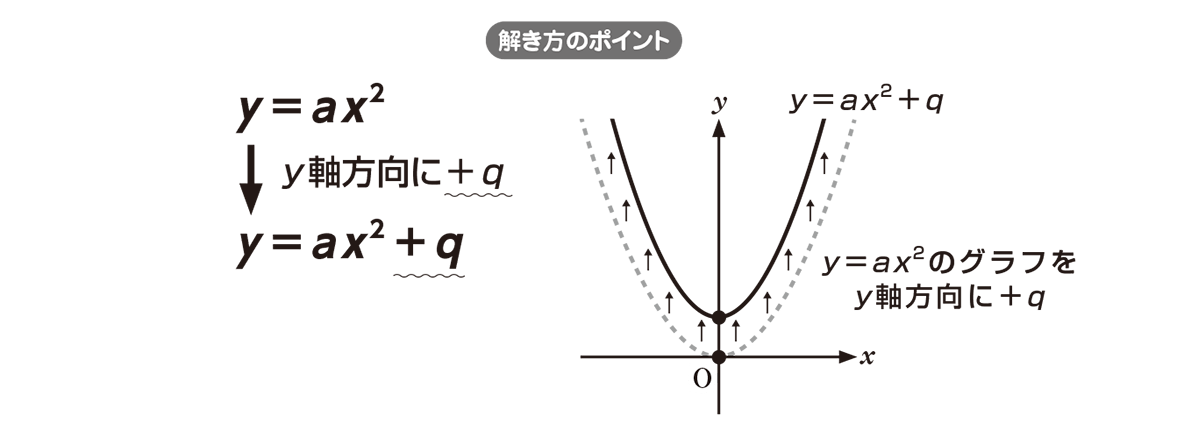



高校数学 Y Ax 2 Qのグラフ2 映像授業のtry It トライイット




The Graphs Of The Two Equations Y A X 2 B X C And Y A X 2 B X C Such That Brainly In
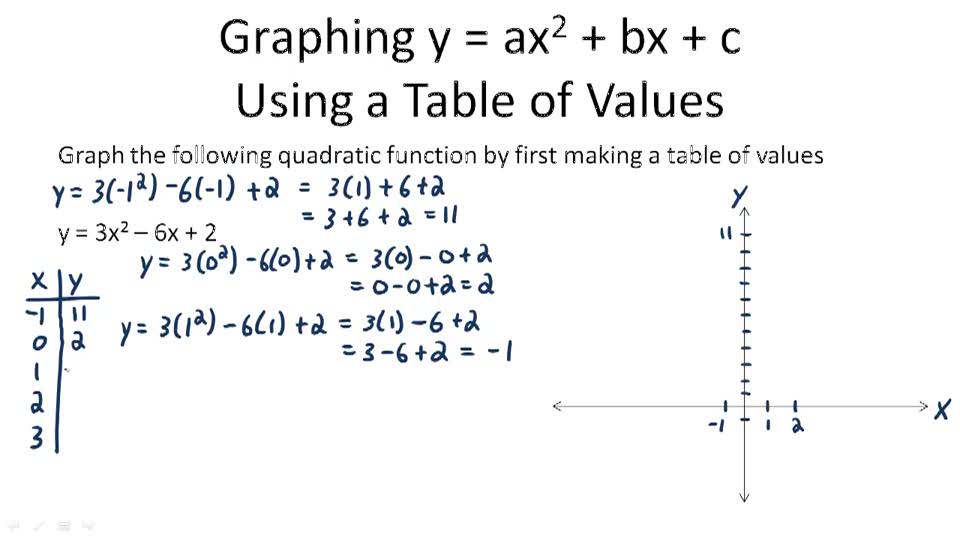



Graphing Quadratic Functions Of The Form Y Ax 2 Bx C Example 1 Video Algebra Ck 12 Foundation



Math 140 Lecture 28 Y Ax2 Bx C University Of San Diego



What Are The Coefficients A B And C Of The Parabola Y Ax 2 Bx C That Passes Through The Point 3 13 And Tangent To The Line 8x Y 15 At 2 1 Quora
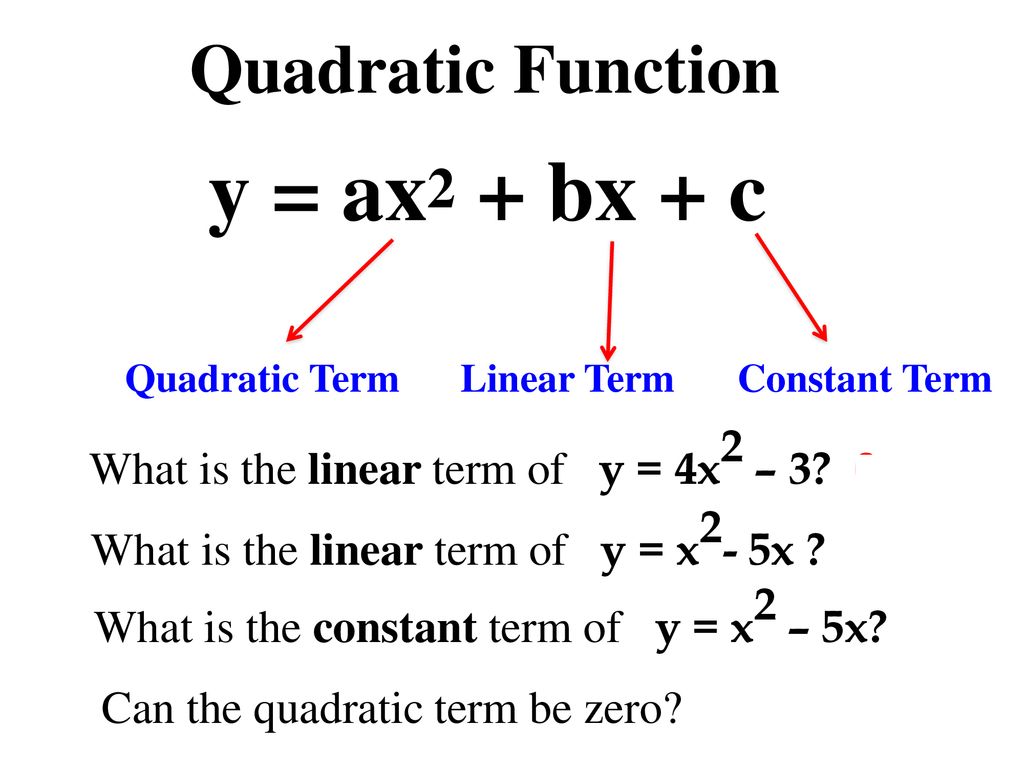



Y Ax2 Bx C Quadratic Function Ppt Download
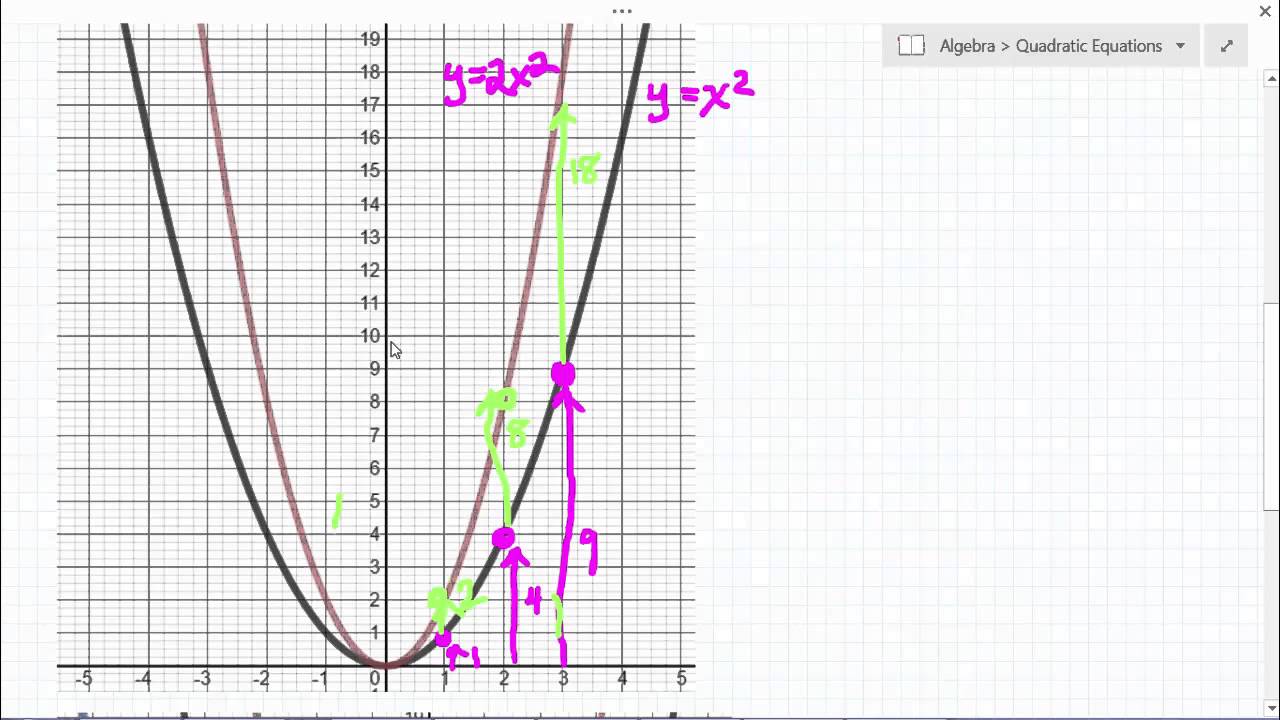



Graphing Parabola Y Ax 2 Youtube




Form Differential Equation For Y Ax 2 B Youtube



Exploring Parabolas Y Ax 2 Bx C




The Diagram Shows The Graph Of Y Ax 2 Bx C Then
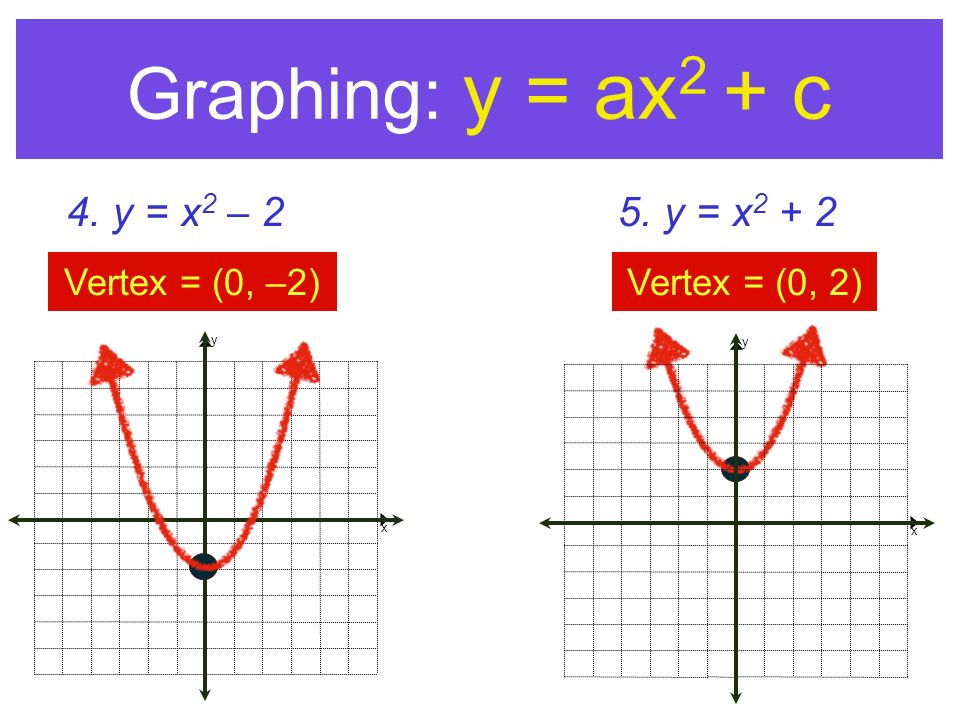



Graphing Quadratics With Vertex And Axis Of Symmetry At The End Of The Period You Will Learn 1 To Compare Parabola By The Coefficient 2 To Find The Ppt Download



トップ100 Yax2bxc Equation 最高のカラーリングのアイデア



Graphing Quadratic Functions Quadratic Functions Have The Form Y Ax 2 Bx C When We Graph Them They Make A Parabola Ppt Download
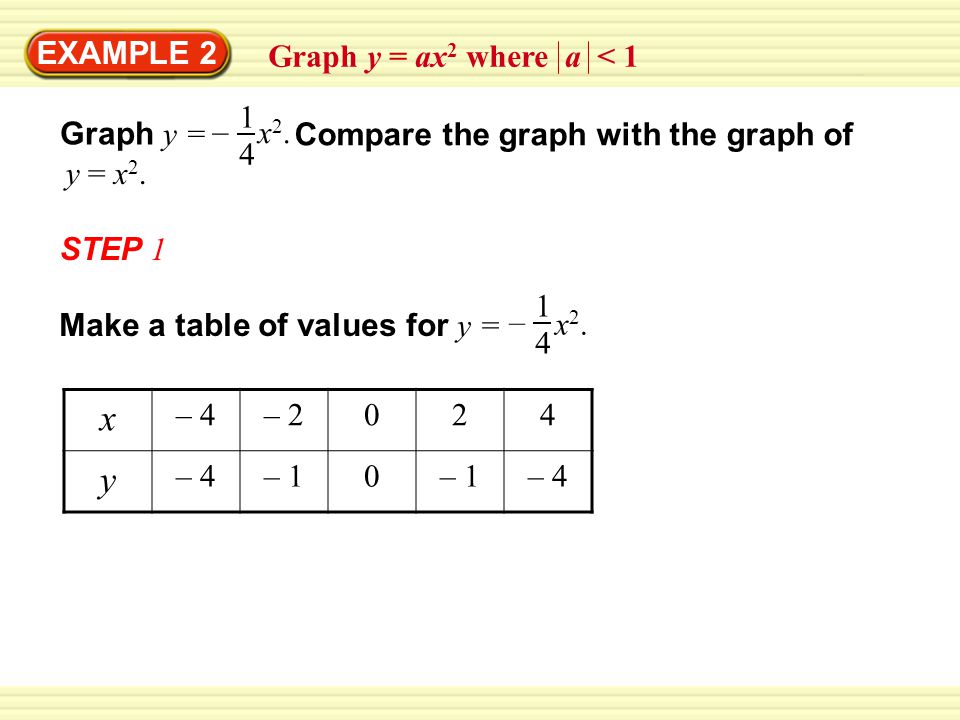



Example 1 Graph Y Ax 2 Where A 1 Step 1 Make A Table Of Values For Y 3x 2 X 2 1012 Y Plot The Points From The Table Step Ppt Download



Form The Differential Equation Having For Its General Solution Y Ax 2 Bx Sarthaks Econnect Largest Online Education Community




Quadratic Function Y Ax 2 Q Geogebra



0 件のコメント:
コメントを投稿